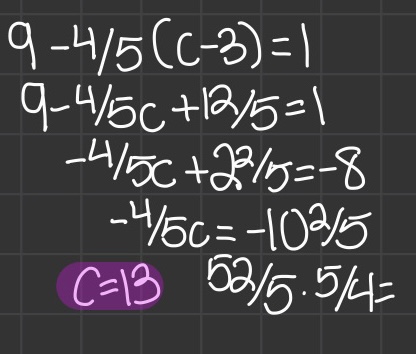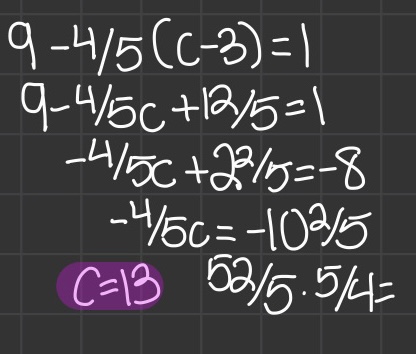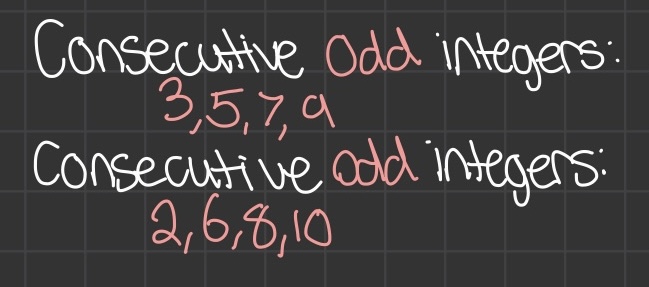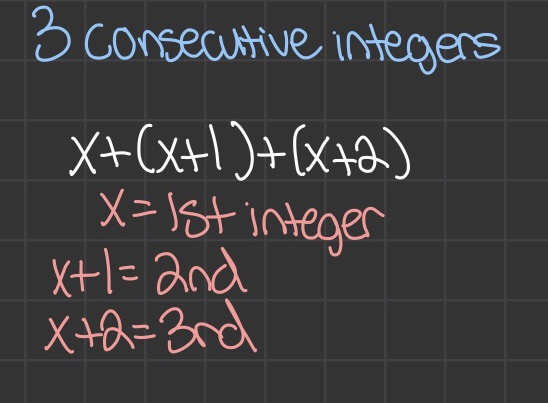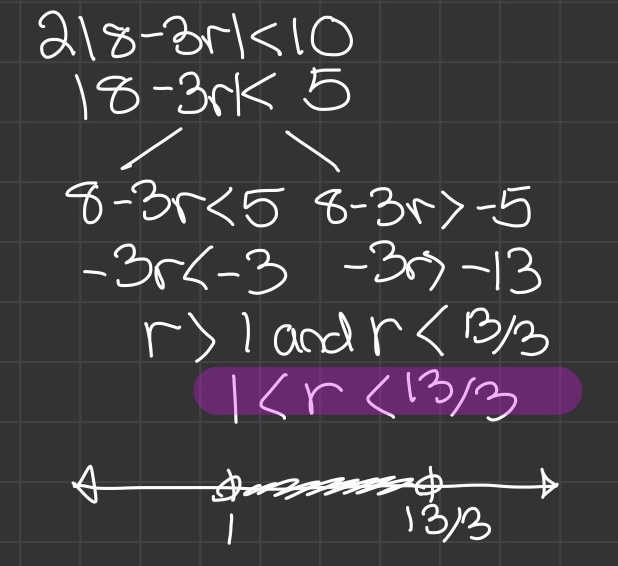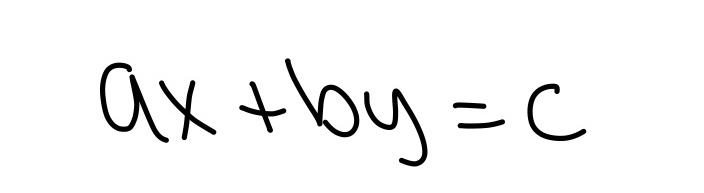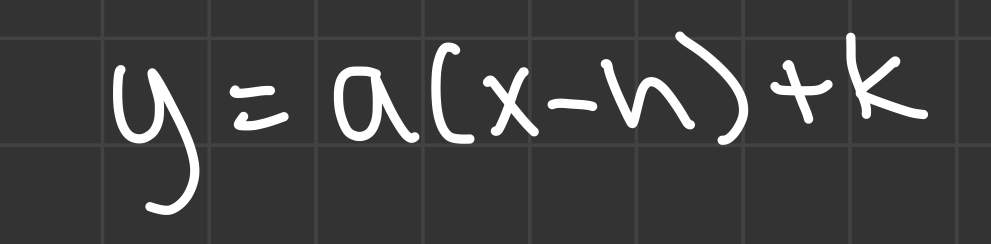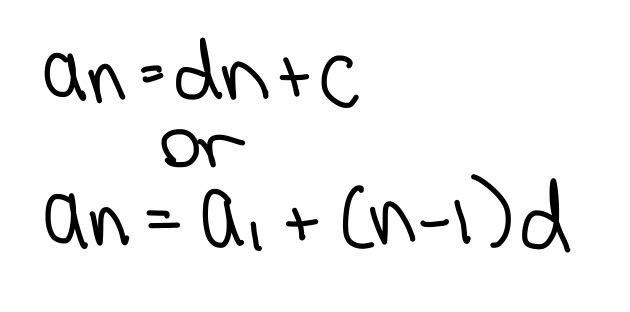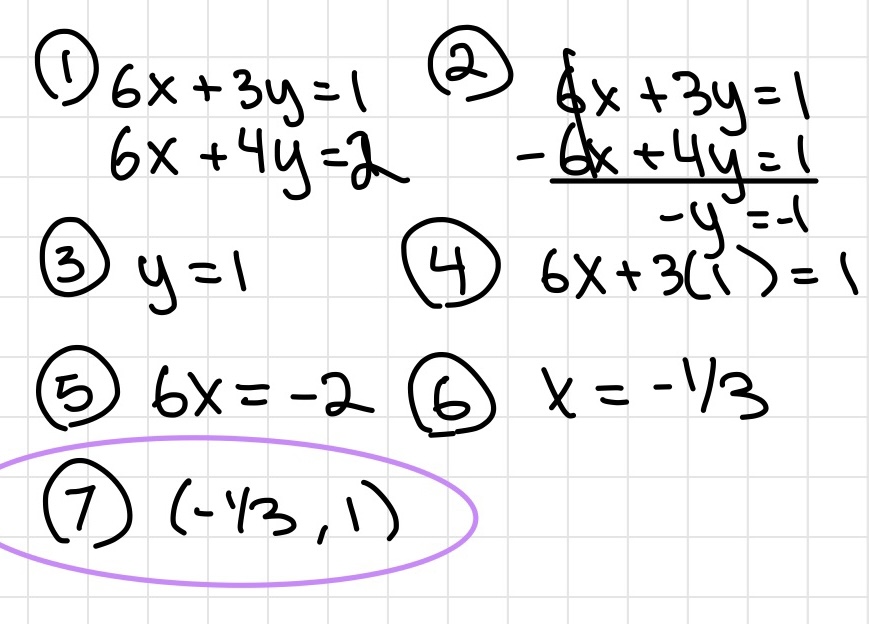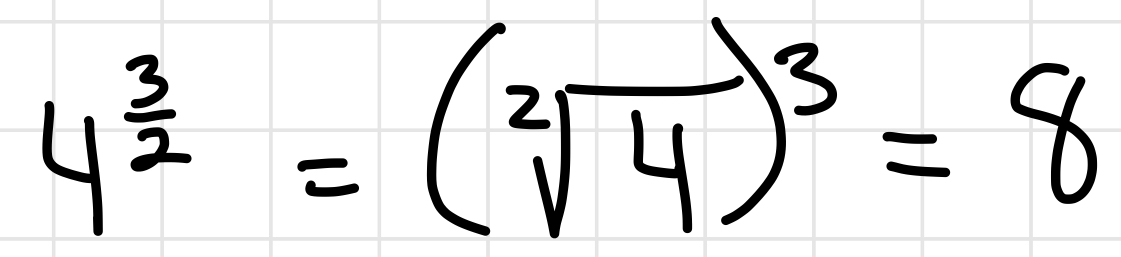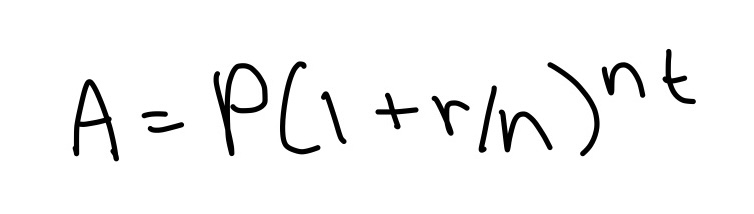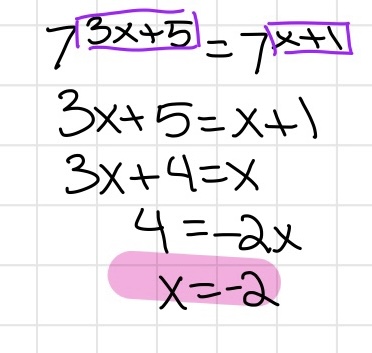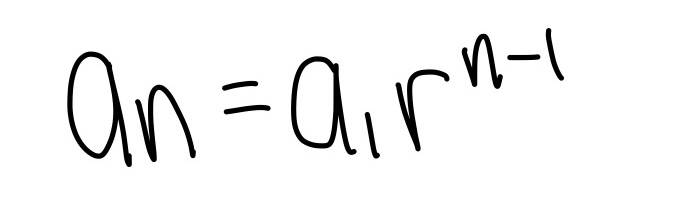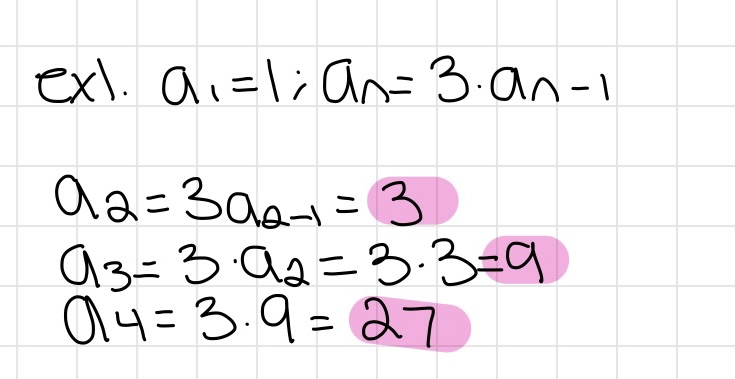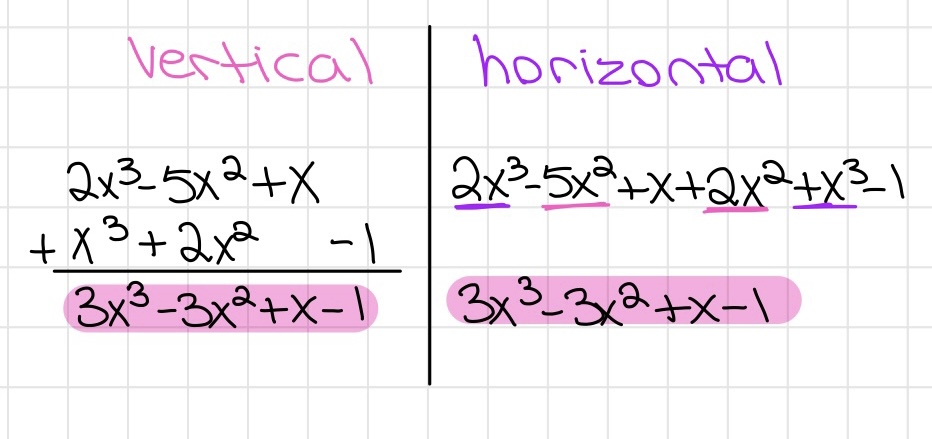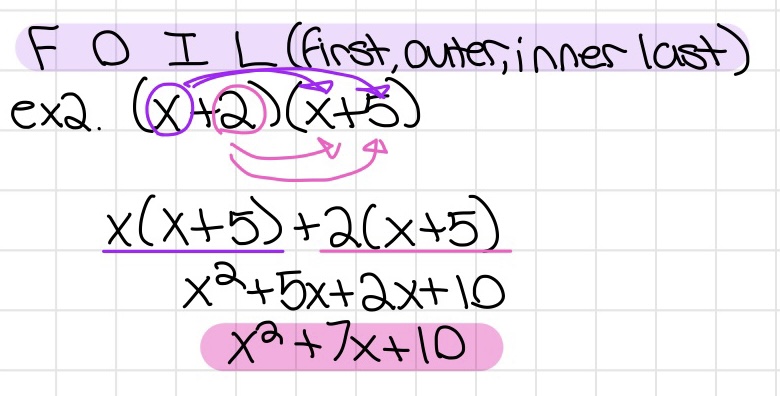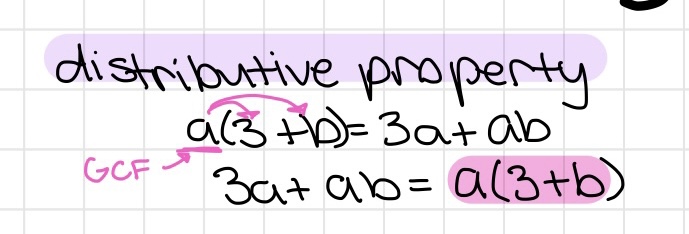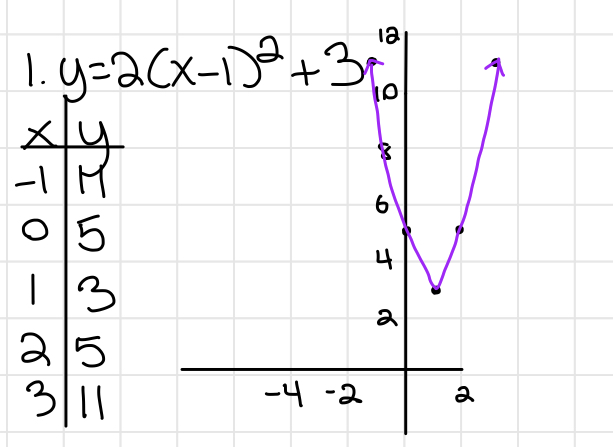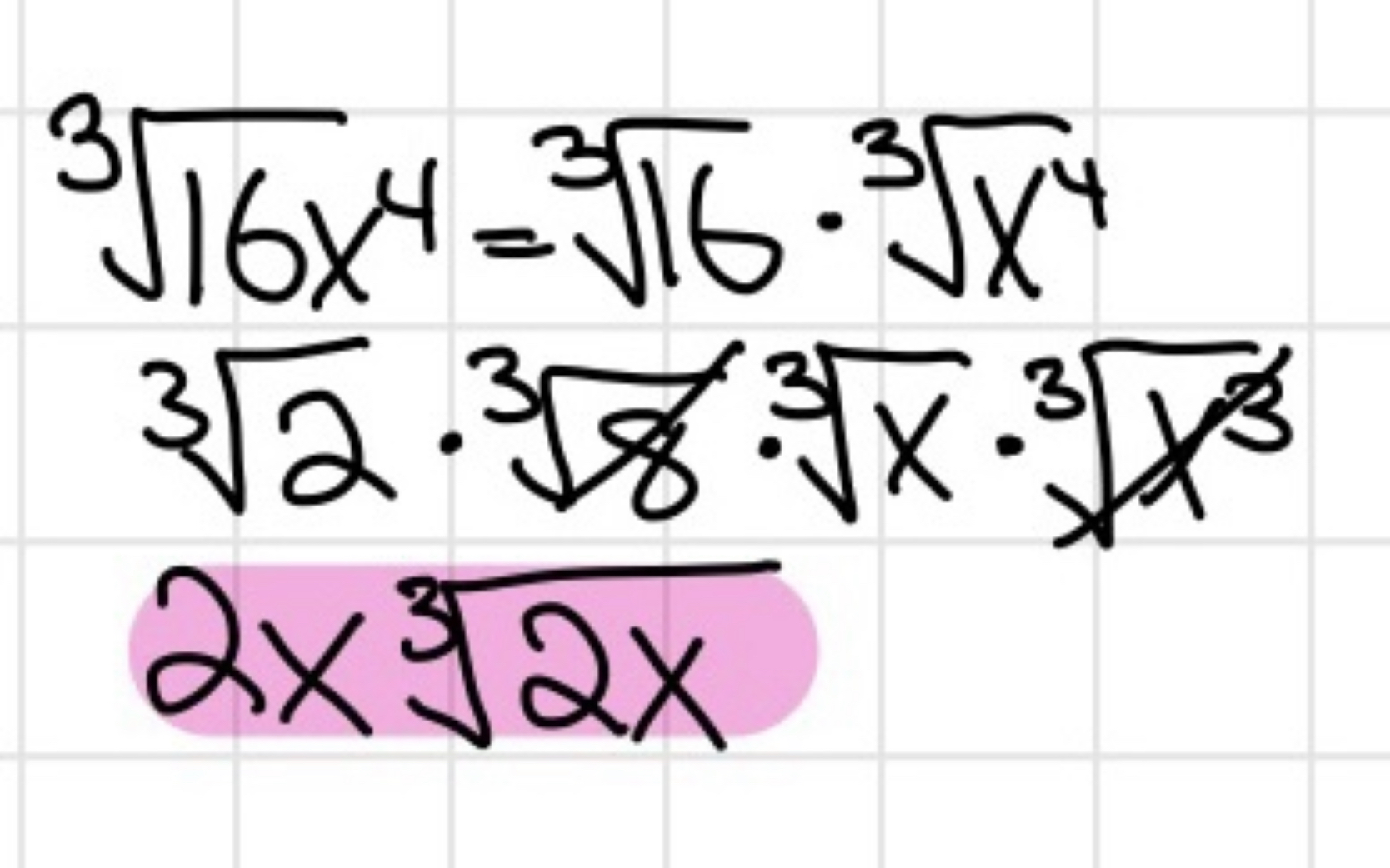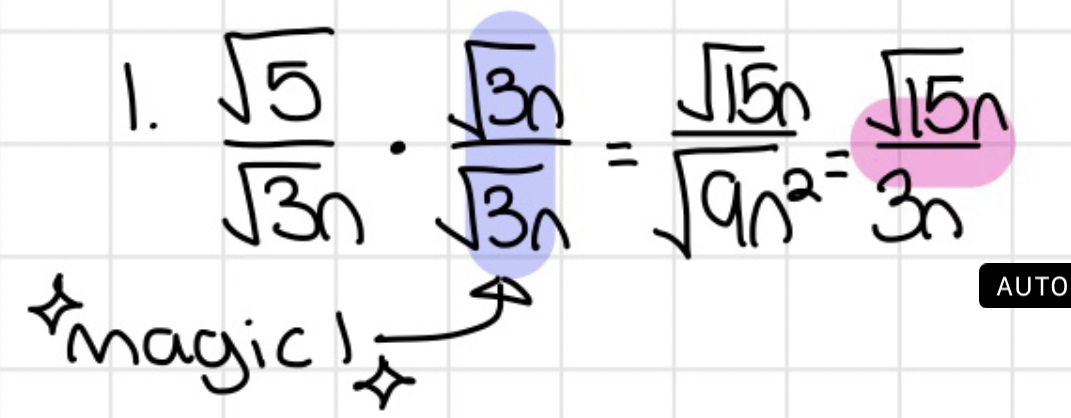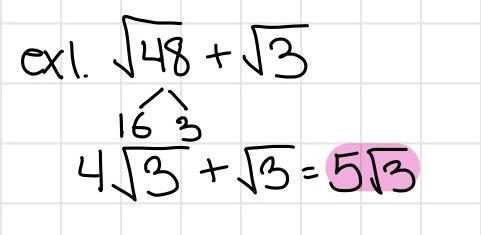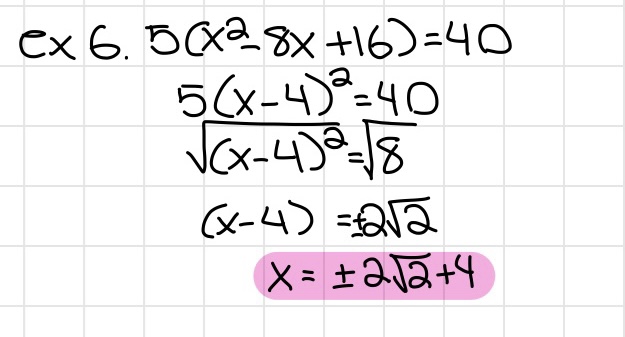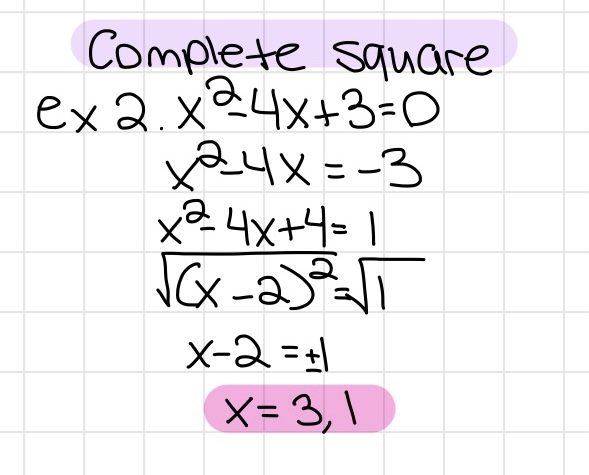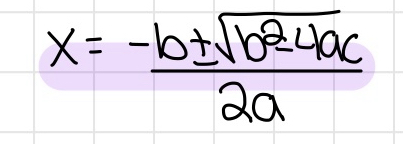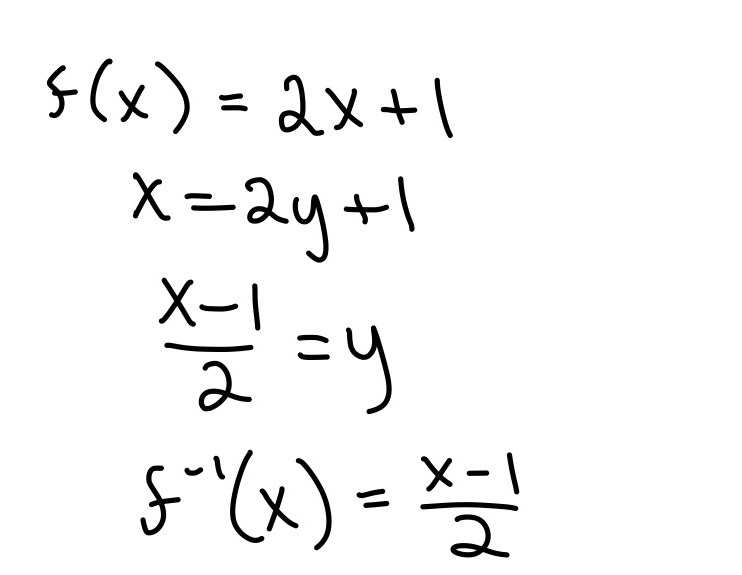~Algebra 1!~

Algebra 1 is the fundamental for every other math course! Having a solid understanding of Algebra 1 will improve your understanding of other math courses significantly

It may seem scary, but it really isn't. We will help you every step of the way!

Solving Multistep Equations

Distribute if necessary
Add/subtract/multiply/divide on both sides so that there is only one of each variable.
Isolate the variable

Solving Multistep Absolute Value Equations

Case of an extraneous solution

|x| = -x, x; |x| ≥ 0
To solve for the other answer, isolate the absolute value sign and make the other side negative
Always check for extraneous solutions: solutions that do not satisfy the equation

Rewriting Literal Equations and Formulas

Literal Equation: an equation with two or more variables
Set the equation to one variable

Consecutive Integers

Consecutive Odd Integers: 3, 5, 7, 9
Consecutive Odd Integers: 2, 6, 8, 10

Consecutive Integer Formulas

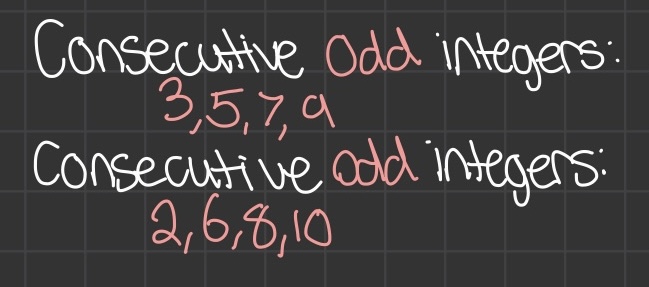
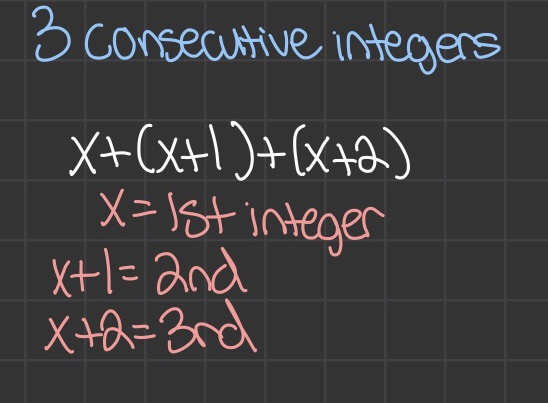
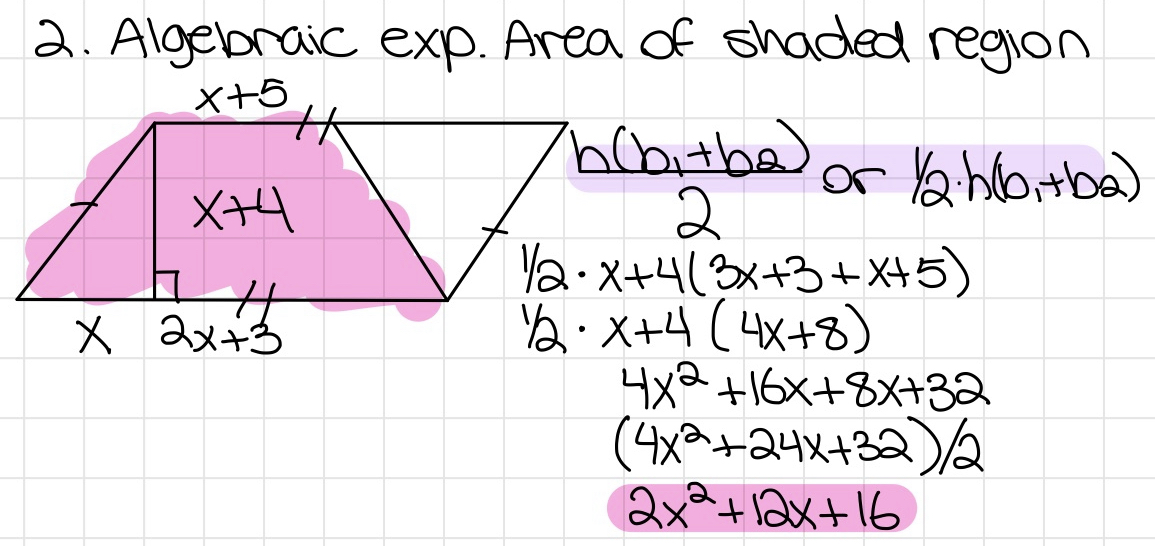
Area and Perimeter

Perimeter: length of the outline of a shape to find the perimeter of a rectangle or any polycom. Add the lengths of each side [2(length + width)]
The area is the amount of pace in a polygon (length · width)
If the sides have variables, don't worry. For perimeter, add all the sides like usual and set it equal to a given perimeter to find the variable. For area, multiply length times width and set it equal to the area to find the variable.

Rate · time = distance (D = rt)

Liquid Mixture Problems

OS = old solution
_ = added solution
NS = new solution
Let x = the added solutions
Amount · acid% = total unit of measurement
Set the old solution equal to the new solution to find x

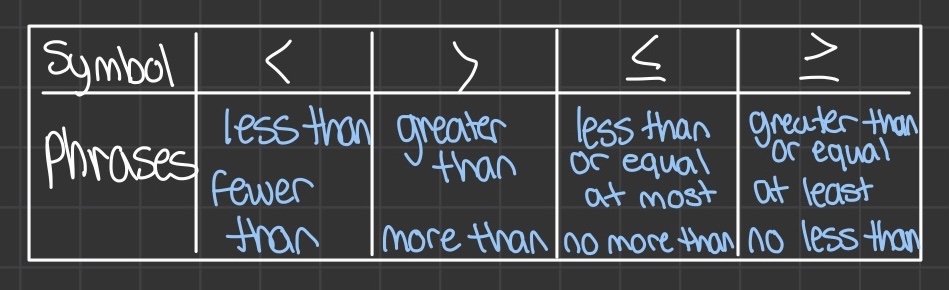
Writing and Graphing Inequalities

Dividing by a negative number on both sides makes the inequality flip

A mathematical sentence that compares expressions
Solving x for an inequality is like solving for x in an equation with one difference: when dividing by a negative number, the sign must flip

Inequalities

Open and Closed Holes/Circle

An open hole/circle represents greater/less than (> <)
An closed hole/circle represents greater/less than or equal to (≥ ≤)

Compound inequalities

And

The value of x is in between two values. x has to satisfy BOTH inequalities
Ex. -2 < x < 3

Or

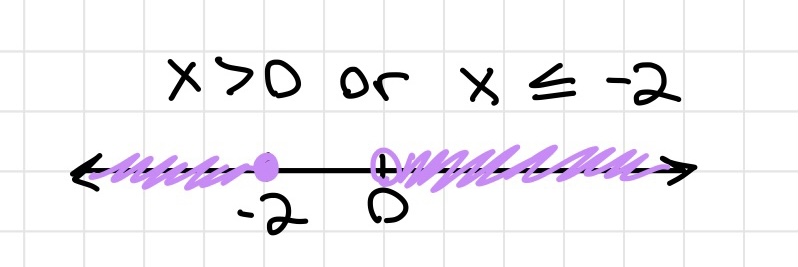
The value of x is NOT in between two values. x has to satisfy EITHER inequality.
Ex. x > 0 or x ≤ -2. x can't be -1 since it is not greater than 0 or less than -2.

Solving Absolute Value Inequalities

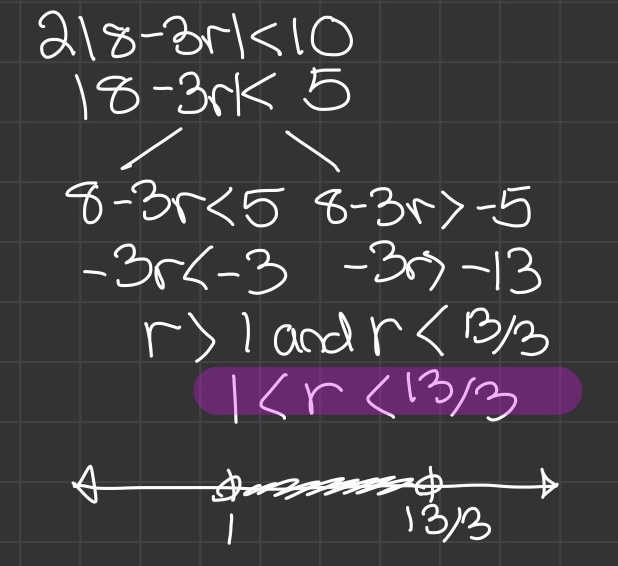
"and" example

"or" example

Solve the inequality for the variable
Solve the inequality again, but this time flip the sign and make one side negative
Find out if the inequality is an "and" or "or" compound inequality
If a variable is less than the SMALLEST number and greater than the LARGEST number, it is an "or" compound inequality
If a variable is less than the LARGEST number and greater than the SMALLEST number, it is an "and" compount inequality

Functions

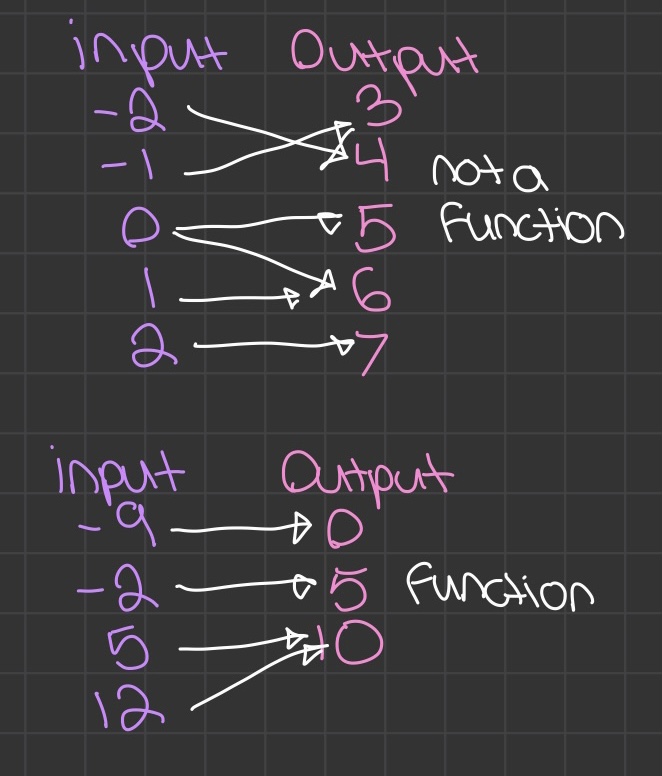
The top example is not a function because there is one input for multiple outputs (does not pass the vertical line test)

A function always has one input and one output only

Relation: pairs inputs with outputs
Represented by ordered pairs or mapping diagram
Function: relation that pairs each input with exactly one output. An input having two outputs is not a function (does not pass the verticle line test)
Vertical line test: tests if a graph is a function. Draw a vertical line directly through the graph. If it touches more than two points on a graph, it's not a function.
Domain = input = x
range = output = y

Linear Functions

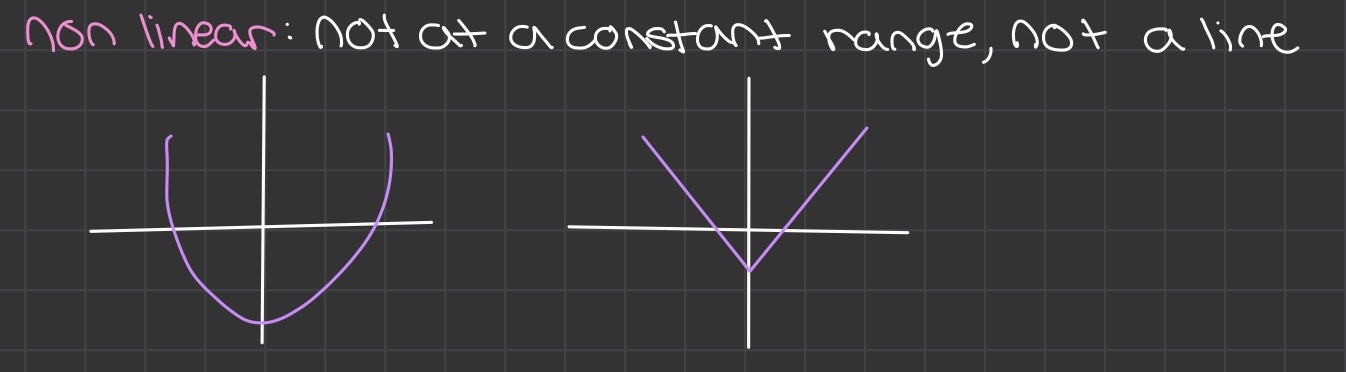
Linear function: non vertical line, constant rate of change, represented with two variables (y = mx + b)
Non linear function: exactly how it sounds. Not a line
Discrete domain: input values of certain numbers
Continuous: input values consist of all numbers in a line

Horizonal and Vertical Lines

y = # is a horizontal line. y will have the same value no matter the x
x = # is a vertical line. x will have the same value. This line is undefined

Function Notation

f(x) = y
Pronounced f of x
f = function
f(x) represents the output of f corresponding to the input of x. Fancy way of saying that there is a y value for every x value.

Graphing Linear Equations in Standard Form

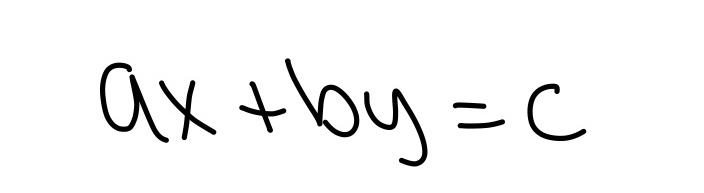
A, B, and C are real numbers; A and B are not 0
Used to find the x and y-intercepts of a graph
Plug 0 into x to find the y-intercept
Plug 0 into y to find the x-intercept
Can convert into slope-intercept form

Graphing Linear Equations in Slope-Intercept Form

m = slope
slope: rate of change; measures the steepness of a line

Find the slope using either these two formulas

(x₁, y₁) (x₂, y₂)
b = y-intercept

Transformations of Linear Equations

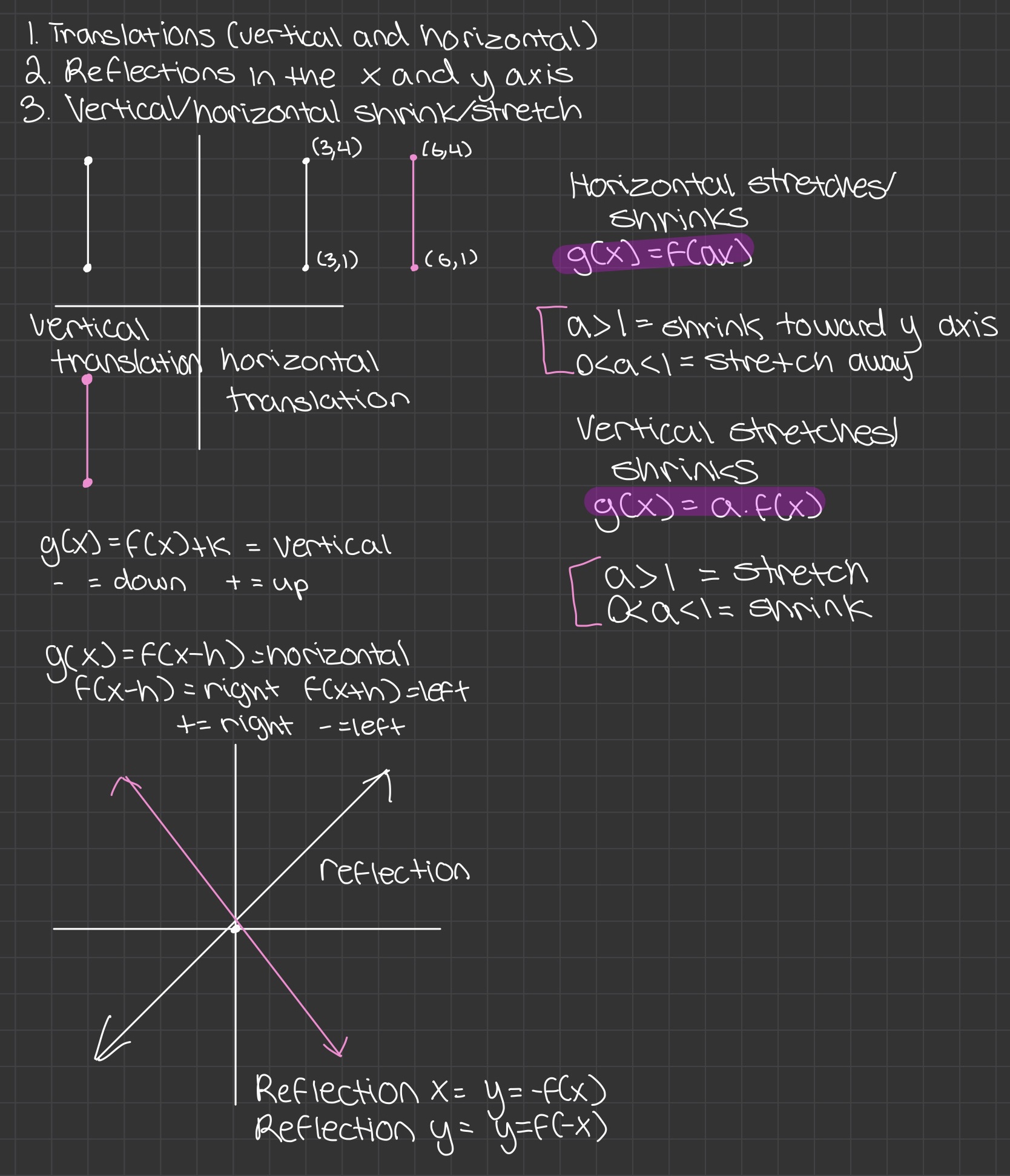
Translations (vertical and horizontal changes of a graph)
Reflections in the x and y axis
Vertical/horizontal shrink/stretch

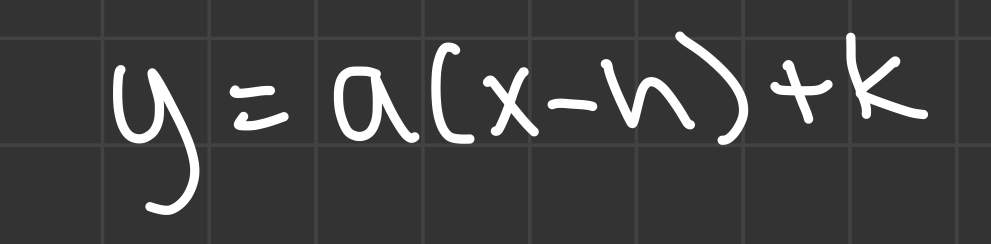
h = horizontal shift. If h is positive (x - h) then the graph shifts to the right. If h is negative (x + h) then the graph shifts to the left
k = vertical shift. A negative k value means the graph shifts downwards. A positive k value means the graph shifts upwards
a = stretch/shrink
g(x) = f(ax): If a > 1 = shrink towards y axis. If 0 < a < 1 = stretch away from the y axis
g(x) = a · f(x): If a > 1 = stretch; becomes steep. If 0 < a < 1 = shrink; becomes shallow
-f(x): the graph reflects across the x axis
f(-x): the graph reflects across the y axis

Writing Equations in Slope/Point-slope Form Parallele and Perpendicular Lines

Can write if given the slope and y-intercept
Find at least two points on a line to graph
Can convert to standard form

The same as Slope-Intercept Form
Use a point (x, y) and the slope to find the slope-intercept form of the slope

Parallel & Perpendicular Lines

Parallel lines

Both slopes have the same slope (m). Only difference is the y-intercept
Ex. y = 2/3x + 1 and y = 2/3x + 4

How to Find the Parallel Line

Create a new slope-intercept equation. Use the variable "b" to replace the y-intercept. This is what will be solved for later
Plug in the given ordered pair and the slope into the equation
Solve for the b value to get the parallel line that passes through a given point

Perpendicular lines

Both slopes have an opposite value and reciprocal of each other
Ex. y = 2/3x + 1 and y = -3/2x - 7

How to Find the Perpendicular Line

Find the reciprocal and negative value of the given slope
Create a new slope-intercept equation. Use the variable "b" to replace the y-intercept. This is what will be solved for later
Plug in the given ordered pair and the new slope into the equation
Solve for the b value to get the perpendicular line that passes through a given point

Arithmetic Sequences

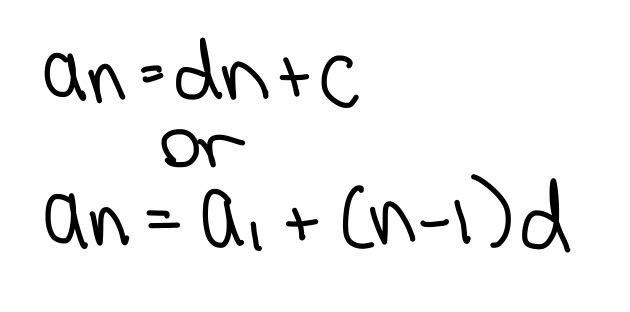
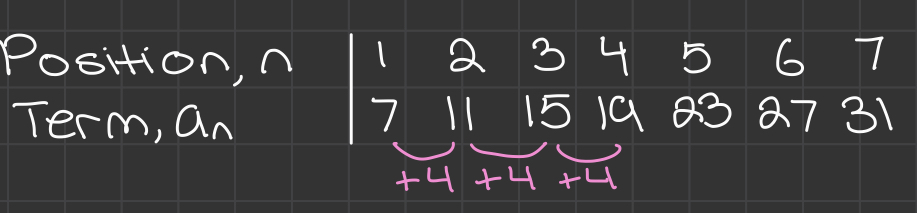
Finds the nth term of a sequence. Has LINEAR nth term rules (addition/subtraction).
d = common difference (pattern in the sequence). Ex. add 6 for each term
an = nth term of the sequence
a₁ = first term of the sequence
The bottom formula is the same as point slope form

Solving System of Linear Equations

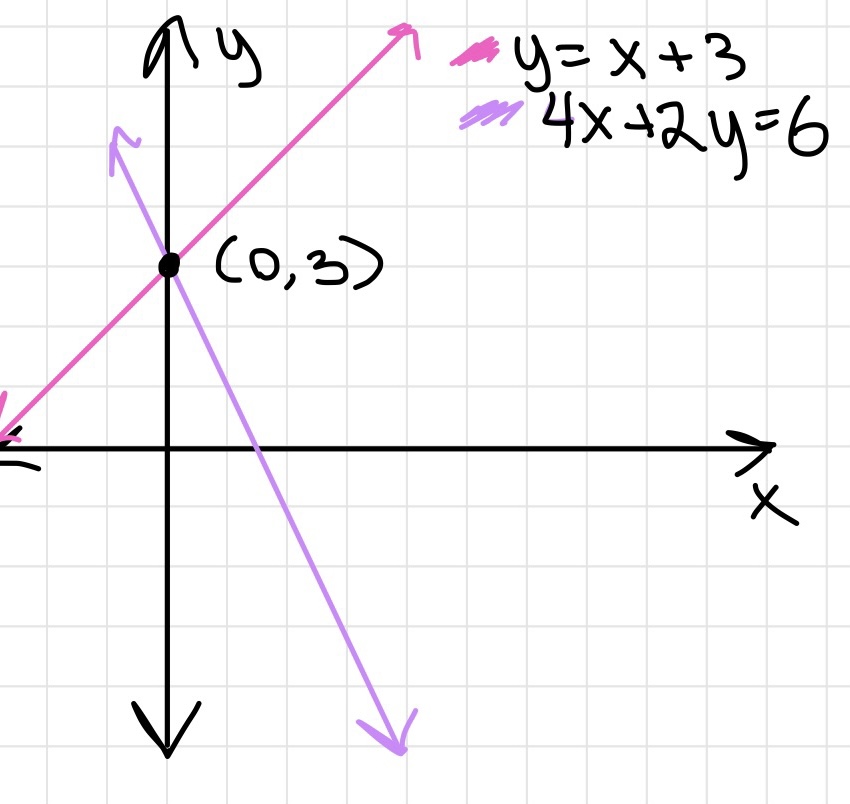
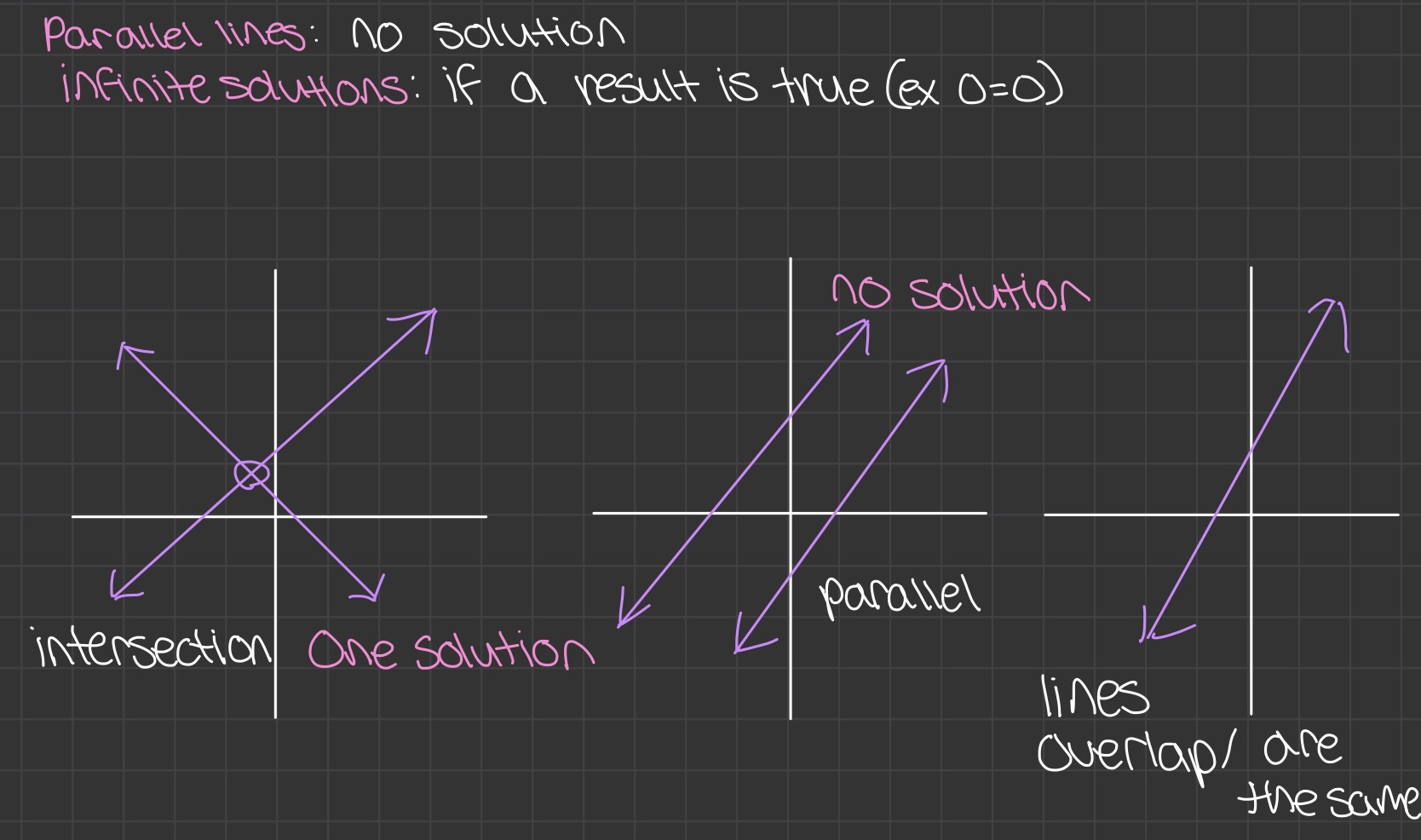
Graphing

The intersection of the linear equations is the answer to the system
Parallel lines = no intersection = no solution
Lines overlap = are the same linear equation = infinitely many solutions

Substitution

Substitute one equation into the other's x or y respectively
Best used when equations in standard form are given

Elimination

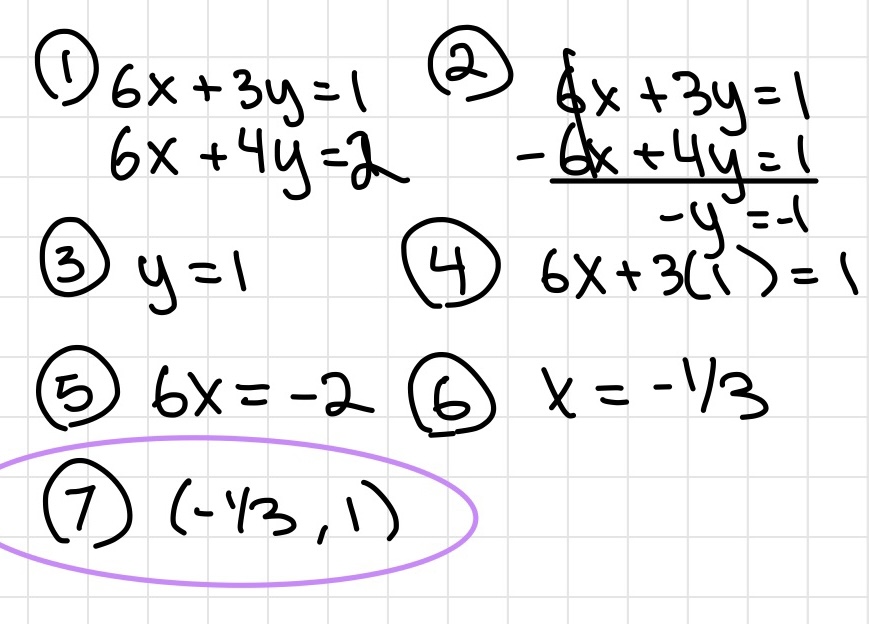
Eliminate one variable to solve for the other by adding or subtracting
Best used when you can eliminate a variable by multiplying one equation with a whole number. Better yet, no multiplication is needed.

Graphing Linear Inequalities

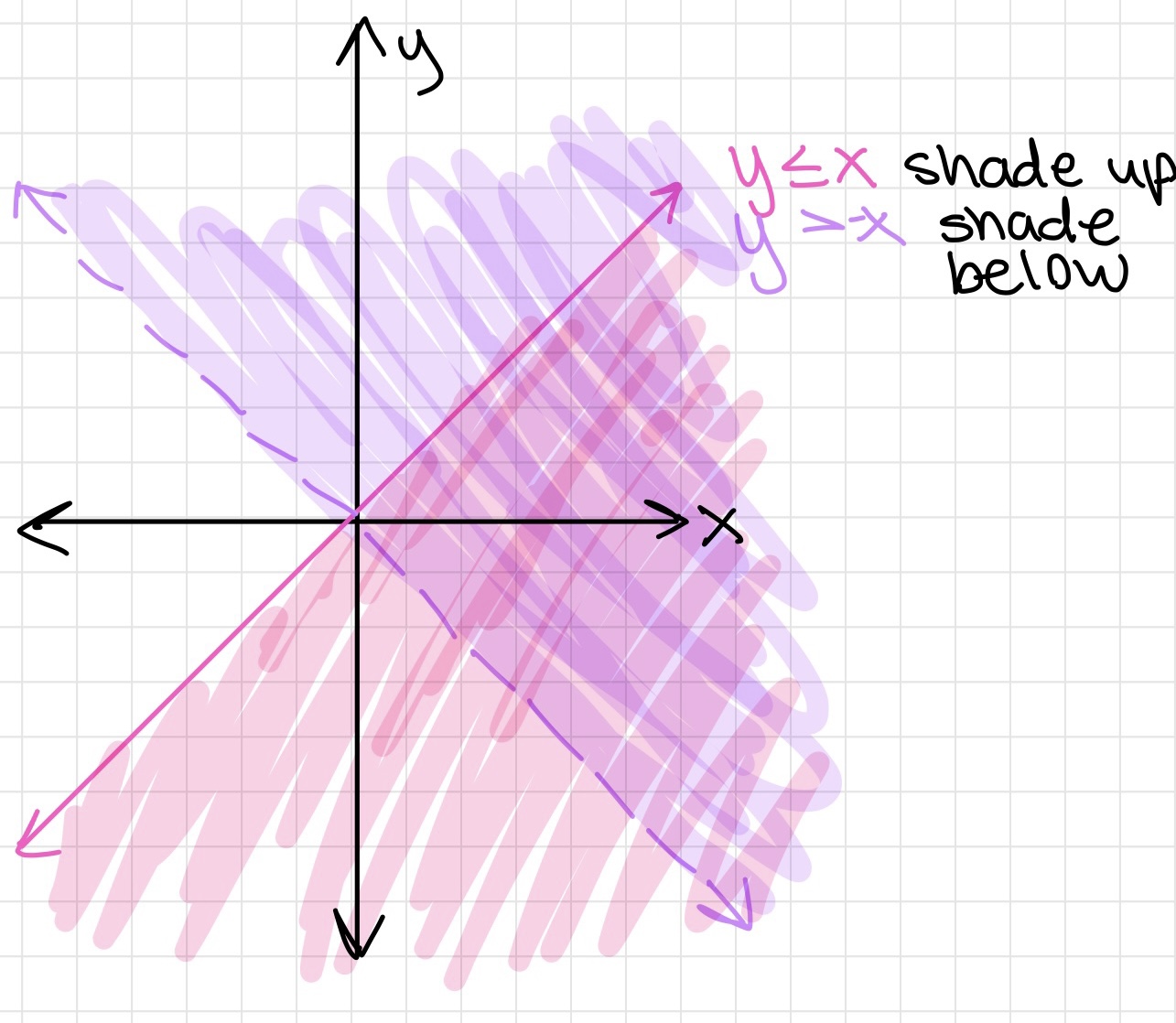
It's messy sorry :(. The peach color represents where the shades intersect

Graph of an inequality on a coordinate plane

Graph like you would a normal linear function
y > x = shade above the line
y < x = shade below the line
Where the shading overlaps = shared values of graphs

Properities of exponents

Product of powers: 2³ · 2² = 2⁵ = 32
Power of a power: (2³)²
Power of a product: (2xy)³ = 8x³y³
Negative exponent: (2/5)^-2 = 25/4. If the numerator has a negative exponent, it becomes the denominator. It is the opposite if the denominator has the negative exponent.
Zero exponent: (20000)⁰ = 1
Quotient of powers: x⁵/x² = x³
Power of a quotient: (x / y) ²

Polynomials

Monomial: a number, variable, or product of both with a non negative exponent (ex. 10, b, 10b, 4ab²)
Binomial: two monomials being added or subtracted (ex. 2x - 3y)
Trinomial: three monomials being added or subtracted (ex. 2x - 3y + 4z)
Polynomial: xy² + x²y + y²-z²

Rational/radical exponents

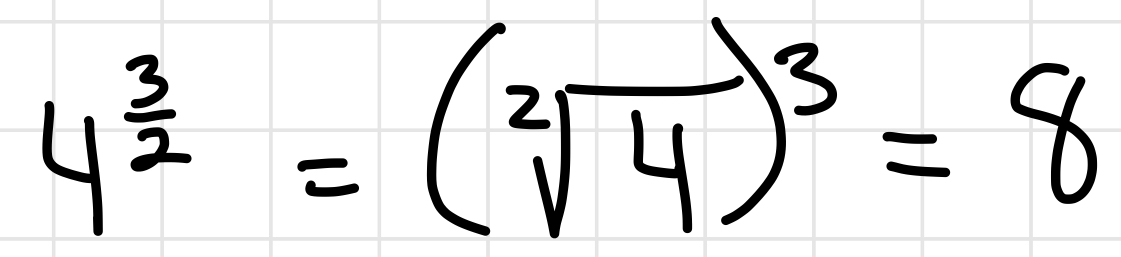
Example of an exponent as a fraction

Rational number: a number that can be written as a fraction
The denominator of a radical exponent is what the root will be (ex. x^1/2= √x
The numerator of a radical exponent is what the number will be raised to the power of (ex. x^3/2= (√x)³

Exponential graph

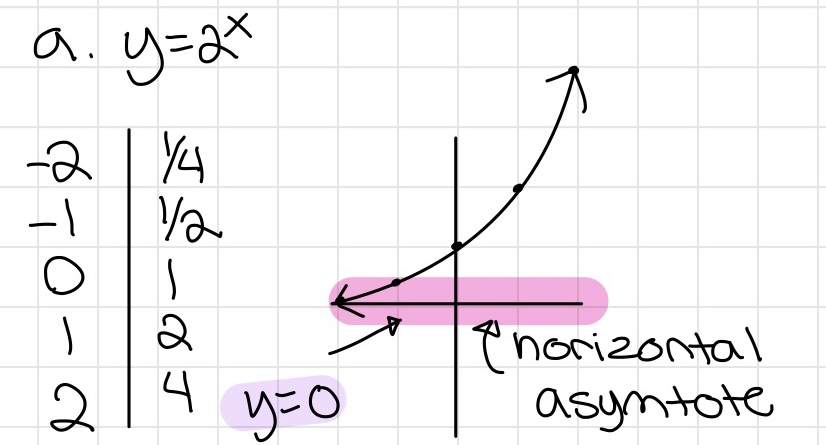
Has a horizontal asymptote: the line graph gets close but never crosses it
Focal point: first integer point closest to HA
a: distance between focal point and horizontal asymptote (+ = focal point is above HA, - = focal point is below HA)

Growth and decay

(1-r) = decay factor
(1+r) = growth factor
Growth: occurs when a quantity is raised by the same factor over equal intervals of time
Decay: occurs when a quantitiy decreases by the same factor

Compound Interest

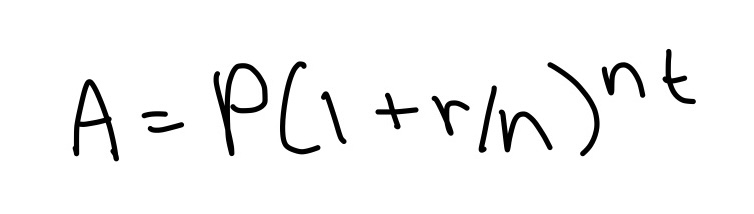
For n compoundings per year

P = principal/initial value for investment
r = annual interest rate
t = years
n = number of compoundings per year

Solving Exponential Equations

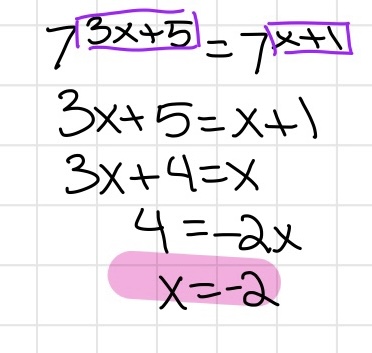
Graphing: this is too tedious please don't graph
Create equal bases: make the base of each side the same to solve
Once the bases are the same, set the exponents equal to each other and solve for x
Equations that are impossible to make the same base are no solution

Geometric Sequences

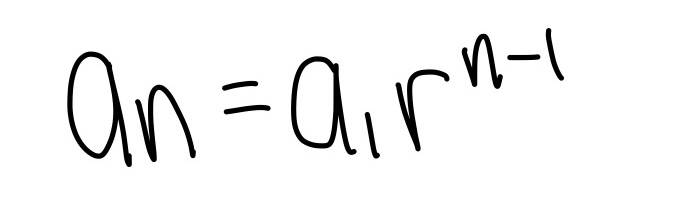
Multiplying sequences
Finds the nth term of a sequence. Has EXPONENTIAL nth term rules (addition/subtraction).
r = common difference (pattern in the sequence). Ex. add 6 for each term

Explicit formula

Recursive Rule

Example of a recursive rule

Finding the term of a sequence

Describes how each term is related to the preceeding term
Given the first term and the recursive rule, find x amount of terms in the sequence
Given a sequence, find the pattern and write it with a recursive rule

Standard Form of Polynomials

Alphabetical order
Exponents decrease from left to right

Adding and Subtracting Polynomials

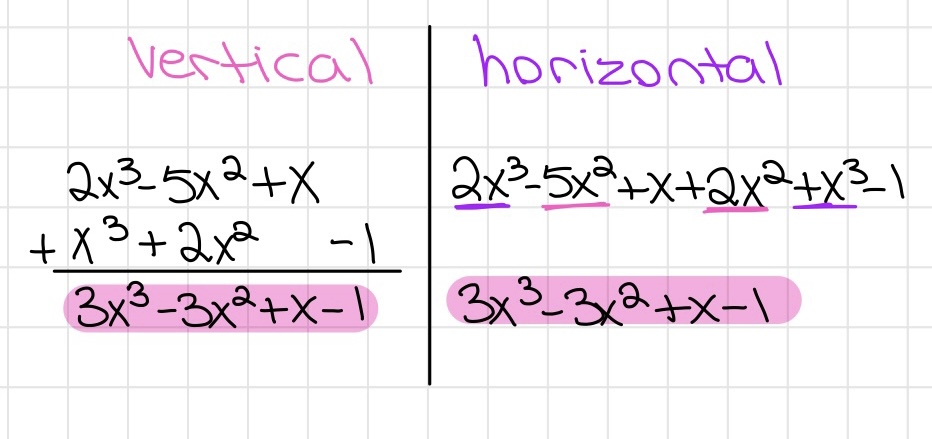
Polynomial degree: the sum of the exponents of the variables in the monomial (largest sum). Ex. 8x³y³ = degree 6
Adding horizontal or vertical is based on preference

FOIL (big word you have probably heard before)

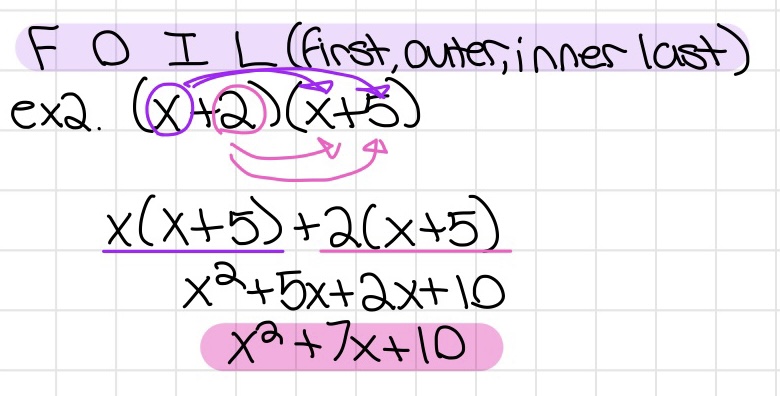
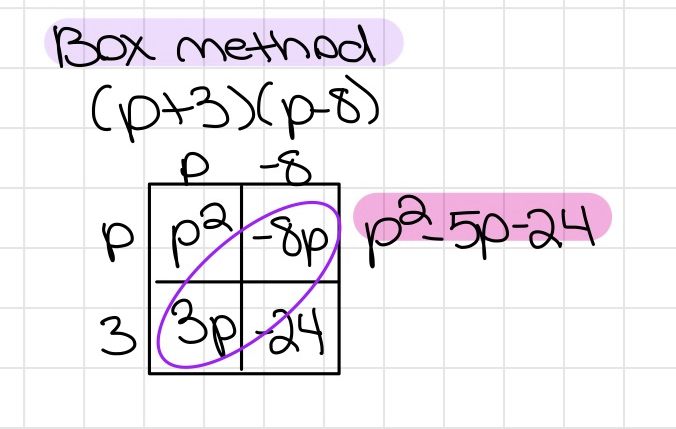
Box method/punnett square-looking method

It is exactly like the distributive property, so don't be nervous ;D
First, outer, inner, last
Box method: it's like a punnett square if you have ever done that

Area and Perimeter

A few notes:

a² + 2ab + b² = (a + b)²
a² - 2ab + b² = (a - b)²
(a + b)(a - b) = a² - b²
(a + b)² IS NOT a² + b²

Basics of Factoring

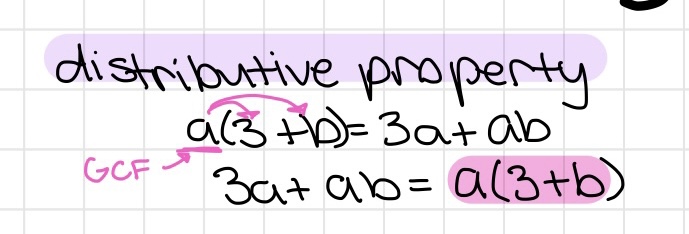
You can take out the greatest common factor out of a polynomial expression and place it outside of a paranthesis like a distributive property
Sometimes, factoring can be used to rewrite an equation
If an equation is equal to 0, the each equation in parenthesis is also set to 0. This can be used to solve for variables

X Box Method for Factoring

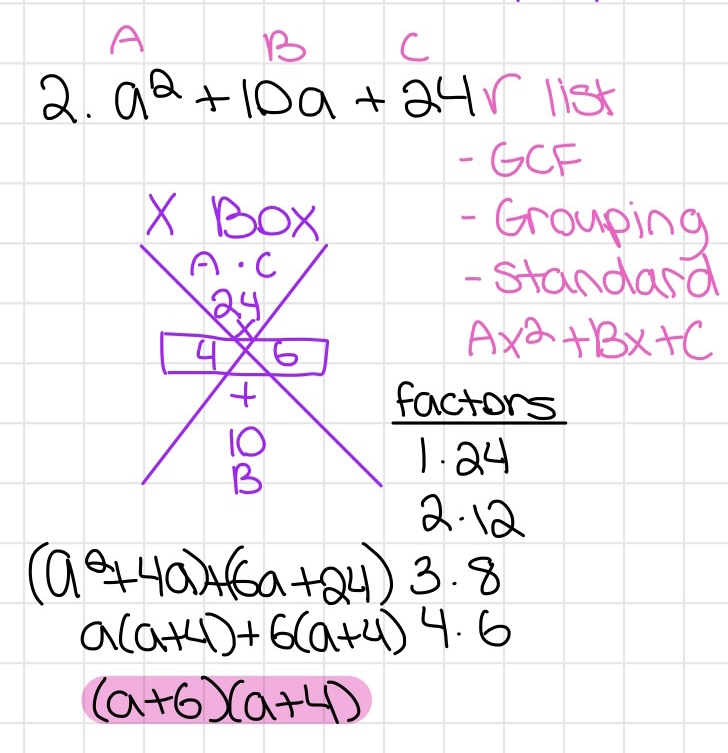
Put "a · c " at the top of the X and "b" at the bottom of the X
Find two numbers that multiply to "a" AND add up to "b"
One they are found, make two binomials like this: (ax² + 1st number)(2nd number + c)
Take out a common factor for each binomial.
If the binomial in the parantheses are the same, which they should be, put the factors into their own binomial in parantheses.

Vertical Motion

S = initial height
v = velocity
t = time

Graphing Quadratics

Quadratic form/standard form: ax² + bx + c
Vertex form: f(x) = a(x - h)² + k
x intercept form: a(x-p)(x-a)

Vertex in quadratic form: (-b/2a), f(-b/(2a))
Vertex in vertex form: (h, k) (when k = y and h = x)
Vertex in x intercept form: V((p+q)/2, f((p+q)/2))

Parabola terms

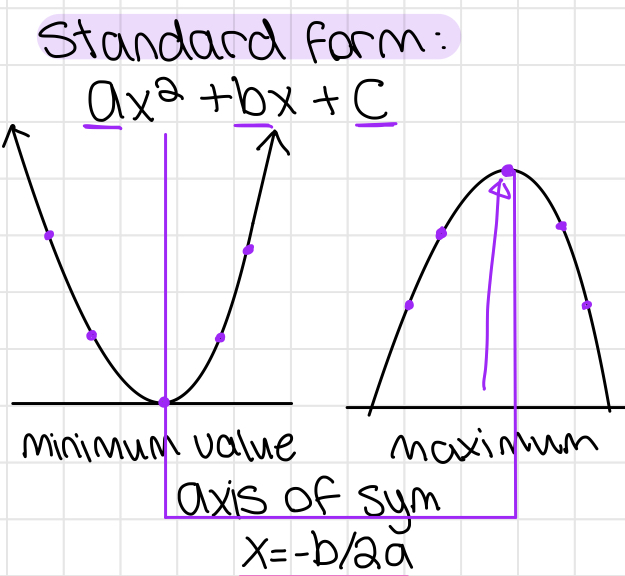
Minimum: the lowest y value of a positive parabola
Maximum: the highest y value of a negative parabola
Axis of symmetry: the x value of the vertex (where the parabola mirrors each side)

Graphing in quadratic/standard form

Find the vertex using the vertex in quadratic form formula
Plug in x values around the vertex and solve for the y values. Those will be ordered pairs on the graph

Graphing in Vertex Form

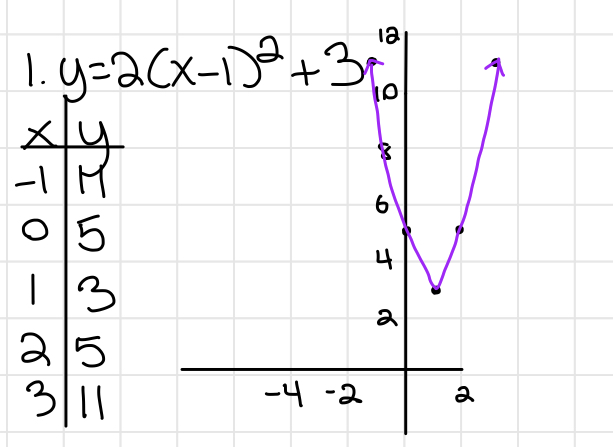
Vertex form: f(x) = a(x - h)² + k
h = horizontal shift. If h is positive (x - h) then the graph shifts to the right. If h is negative (x + h) then the graph shifts to the left
k = vertical shift. A negative k value means the graph shifts downwards. A positive k value means the graph shifts upwards
a = stretch/shrink
-f(x): the graph reflects across the x axis
f(-x): the graph reflects across the y axis

Graphing in X Intercept Form

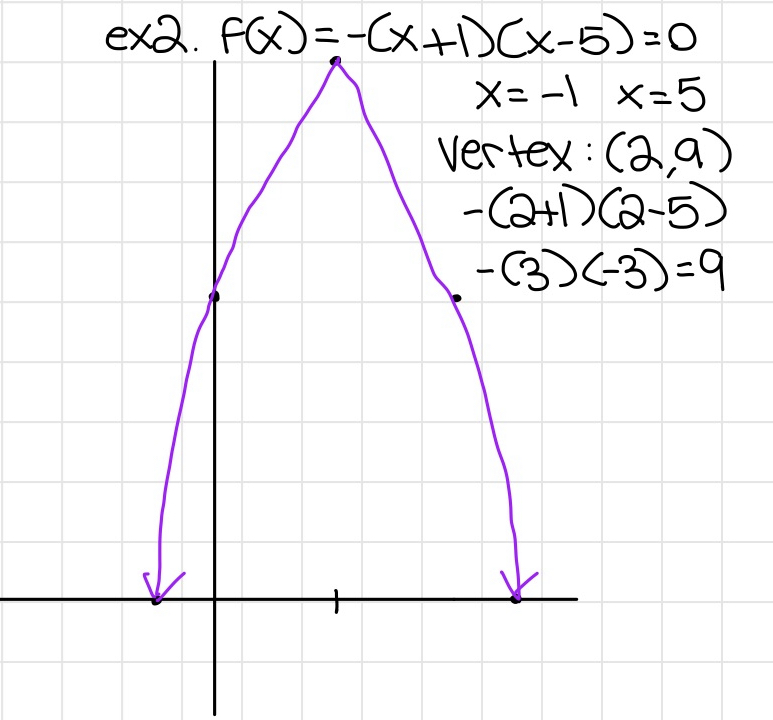
Set the equation to 0
Solve for the x values. These x values are the x intercepts for the parabola
Plot the x intercepts on the graph
The middle x value between the x intercepts is the x value for the intercept
Plug in the middle x value into the equation to get the y value of the vertex
Plot the vertex and connect the dots :D

Simplifying Radical Expressions

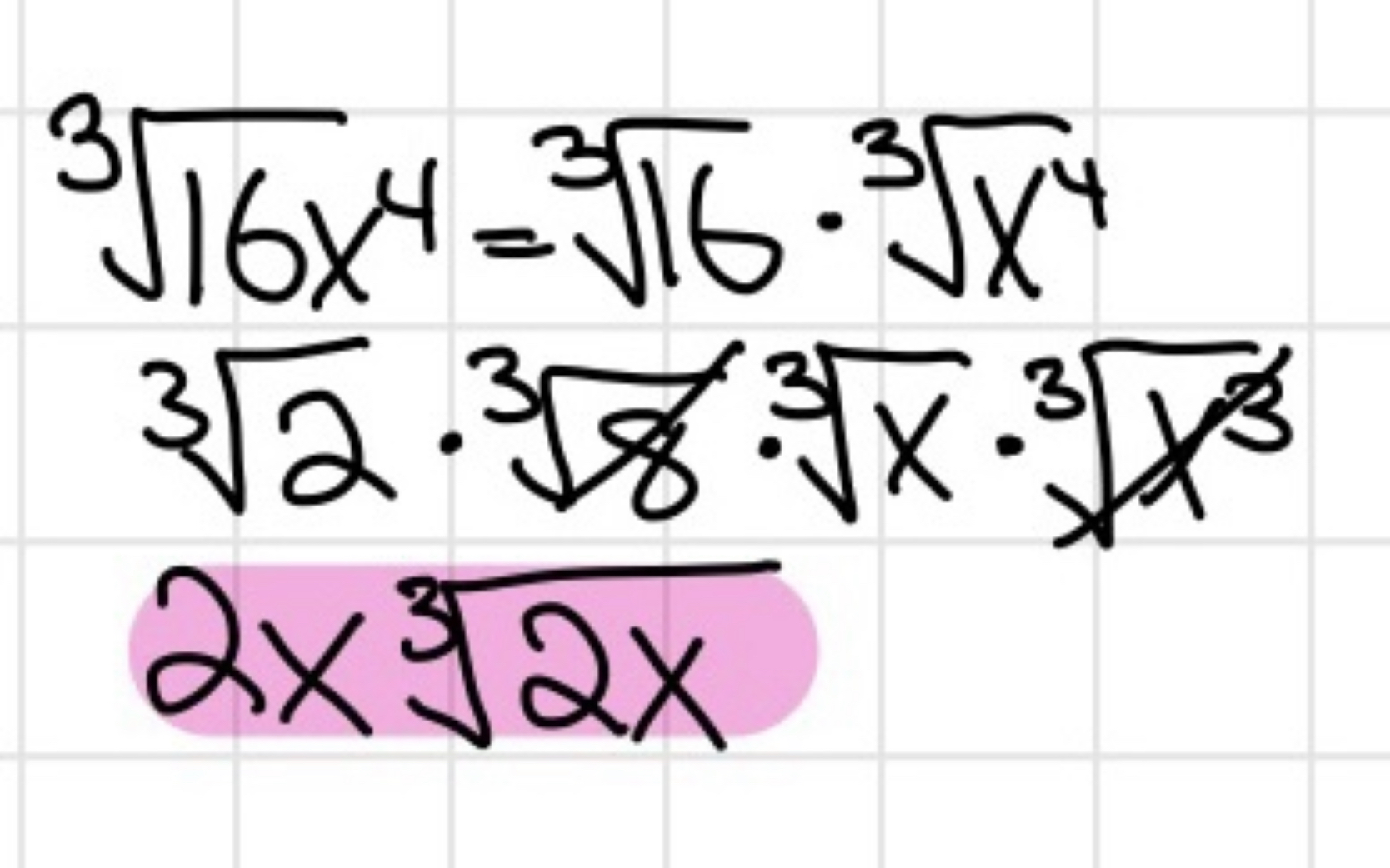
Check to see if the radicand can be split up to a multiplication expression that can be taken the root of (ex. 4, 9, 16, etc. can be square rooted)
If it can, take the root of that first and bring it to the outside of the radical expression.
If there is a variable to the power of something, split it up to a multiplication expression that has a power that can be take the root of (ex. a⁴ can be square rooted to a²

Rationalizing the Denominator

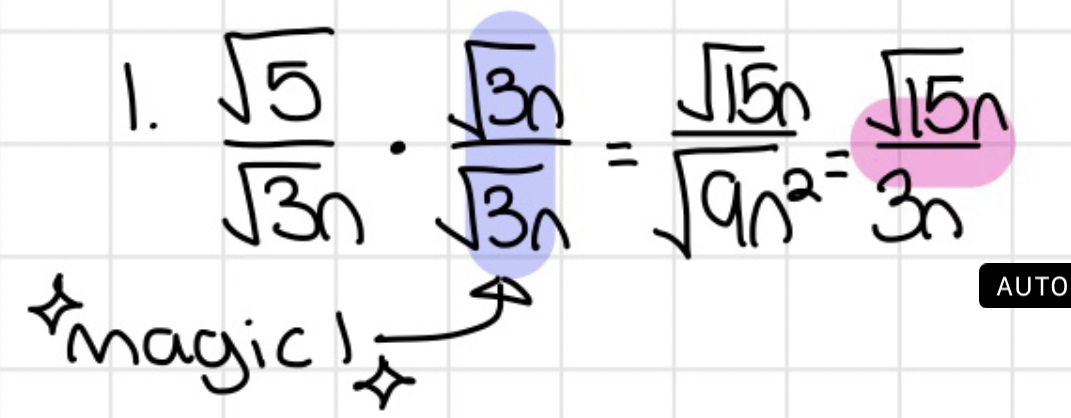
Until you get to higher levels of math, if there is a radical expression in the denominator, you should rationalize it
Multiply the fraction by the denominator over the denominator (ex. √3/√3). This works because you are technically multiplying by 1
Simplify the rest using the techniques in "Simplifying Radical Expressions

Rationalizing the Denominator with a Binomial Radical Expression

To rationalize a denominator like √3 + 2, multiply by the denominator over the denominator BUT CHANGE THE SIGN (√3 - 2)
This makes the denominator into a rational number. Remember this still works because √3 - 2/√3 - 2 = 1

Operations with Radicals

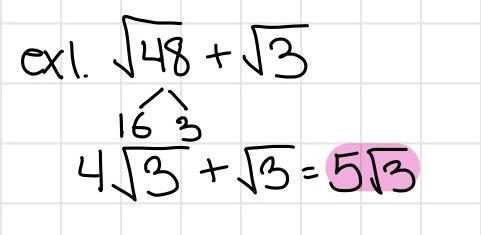
Important: the radicand must be the same in order to add or subtract
This is similar to how the denominator must be the same to add or subtract

Put it all together!

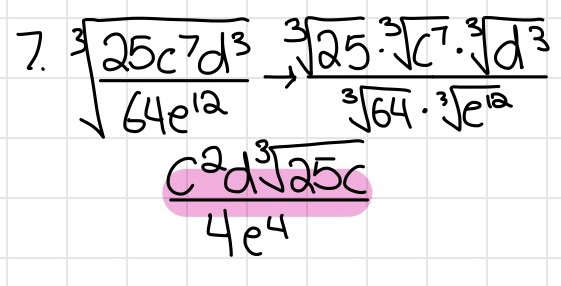
Remember that PEMDAS still applies
Don't be scared of bigger equations with addition, subtraction, multiplication, division, etc. Use what you have learned

Solving Quadratics Using Square Roots/powers

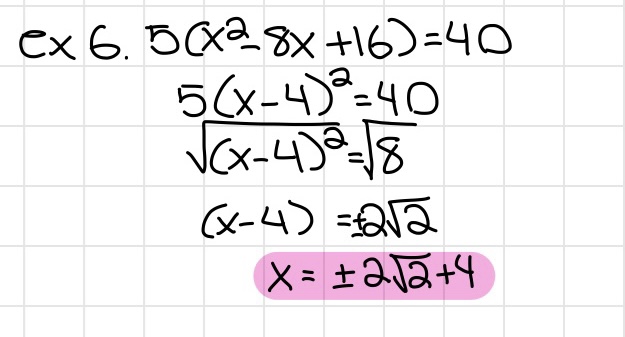
You can take the square root of both sides similar to how you would divide by both sides to find the value of x
You can also take the power of both sides to cancel out a root

Completing the Square

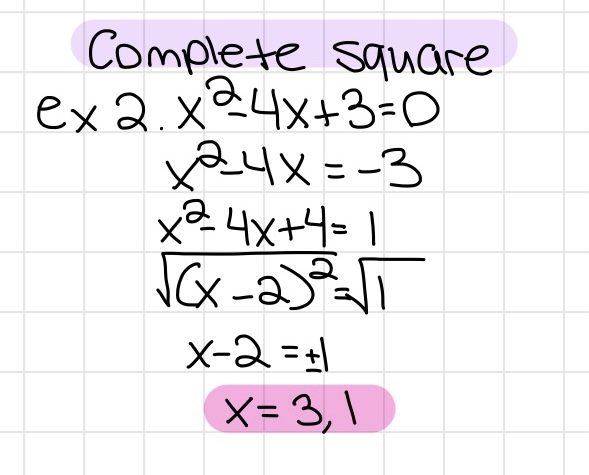
If factoring is too difficult, completing the square may be more efficent

Subtract the constant from both sides
Divide the middle coefficient by 2 then square it. This will be the new constant for the quadratic equation
Remember to add the new constant to BOTH SIDES
Turn the quadratic equation into a perfect square binomial
Take the square of both sides
DONT FORGET THE +/-
Solve for x

Quadratic Formula

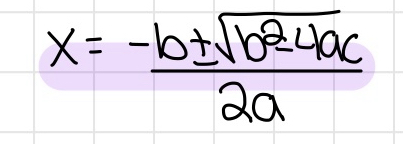
Used to find the zeros of the binomials
When a trinomial isn't factorable, use the quadratic formula to get the exact answers

Discriminant

b² - 4ac
Tells how many x intercepts the graph has
A positive value means there are two x intercepts
A negative value means there are 0 x intercepts (no solution)
A value of 0 means there is only one x intercept (the vertex)

Recap. Different Ways to Solve Quadratics

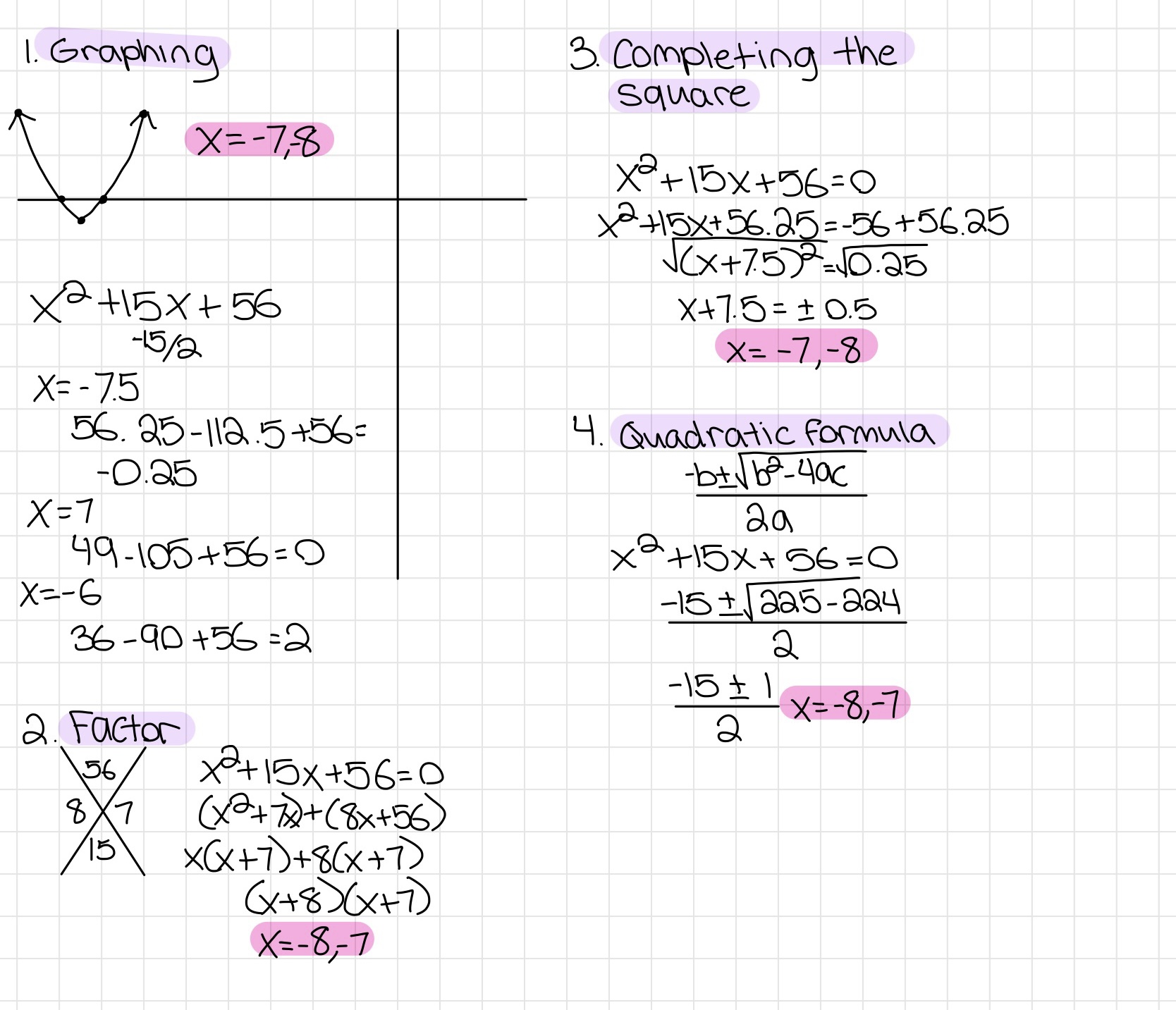
Piecewise functions

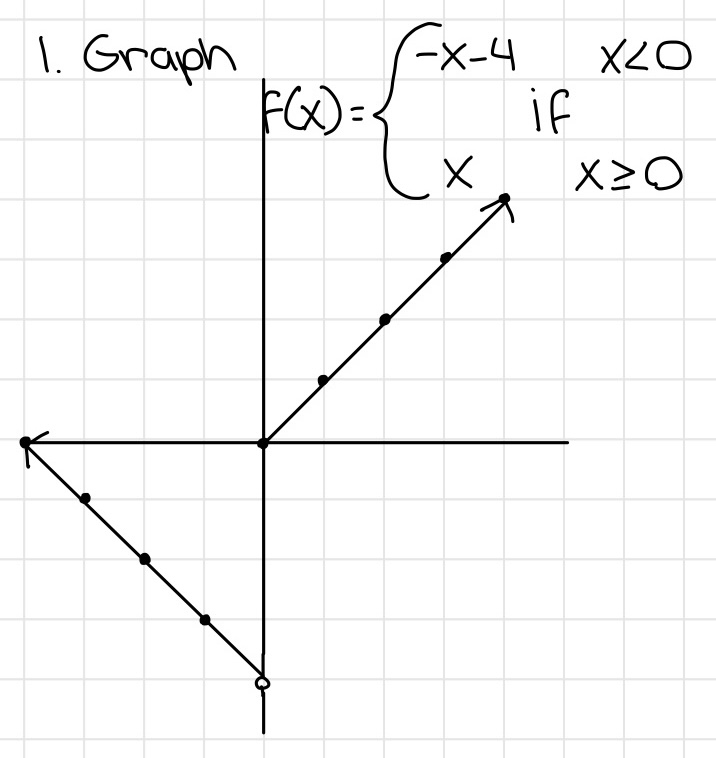
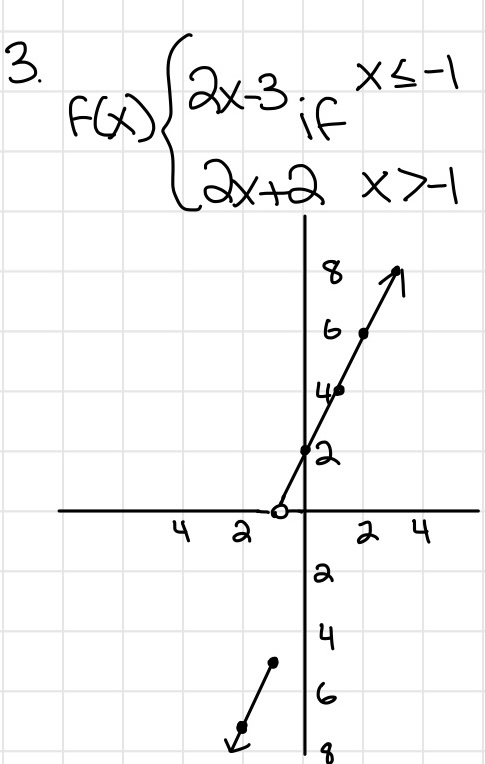
A function that has multiple graphs in it with domain restrictions
Graph each function regularly without domain restrictions, then erase the areas that exceed them
Remember inequalities and open circles

Graphing Square Root Functions

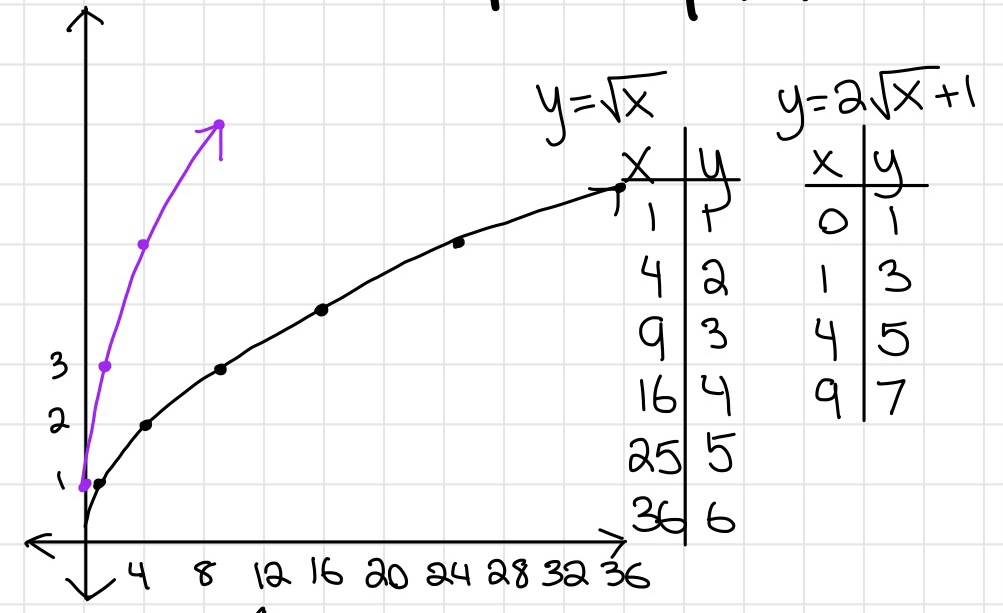
Another transformation, yay! You must love them by now

The same rules apply, however, the radicand CANT be negative EVER

h = horizontal shift. If h is positive (x - h) then the graph shifts to the right. If h is negative (x + h) then the graph shifts to the left
k = vertical shift. A negative k value means the graph shifts downwards. A positive k value means the graph shifts upwards
a = stretch/shrink
g(x) = f(ax): If a > 1 = shrink towards y axis. If 0 < a < 1 = stretch away from the y axis
g(x) = a · f(x): If a > 1 = stretch; becomes steep. If 0 < a < 1 = shrink; becomes shallow
-f(x): the graph reflects across the x axis
f(-x): the graph reflects across the y axis

Graphing Cube Root Functions

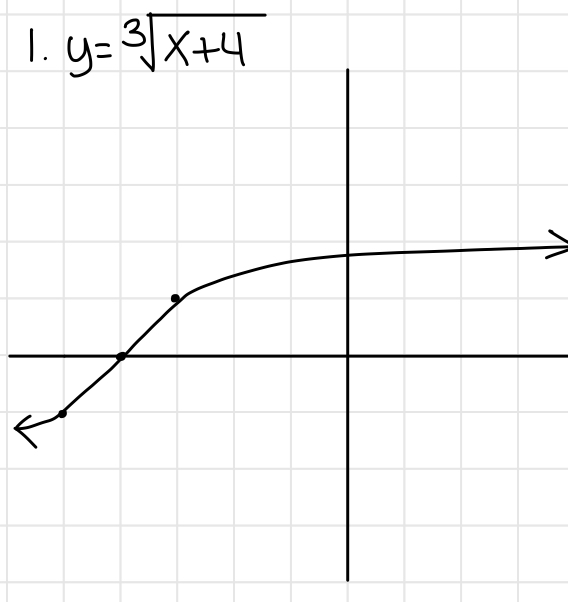
It's similar to graphing square root functions, however, the radican can be negative now

Inverse of a function

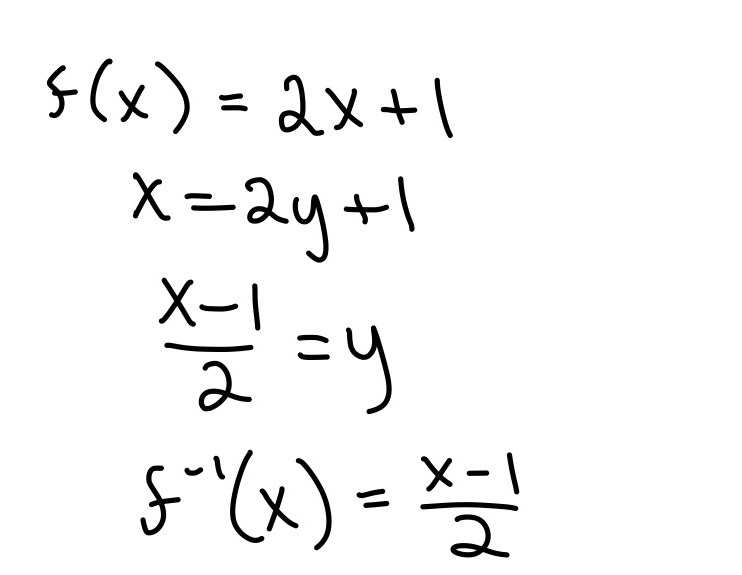
Inverse Functions

IS NOT 1/f
Swap x and y then solve for y to find the inverse function
If you are trying to find the inverse of a parabola, make sure to find the restriction