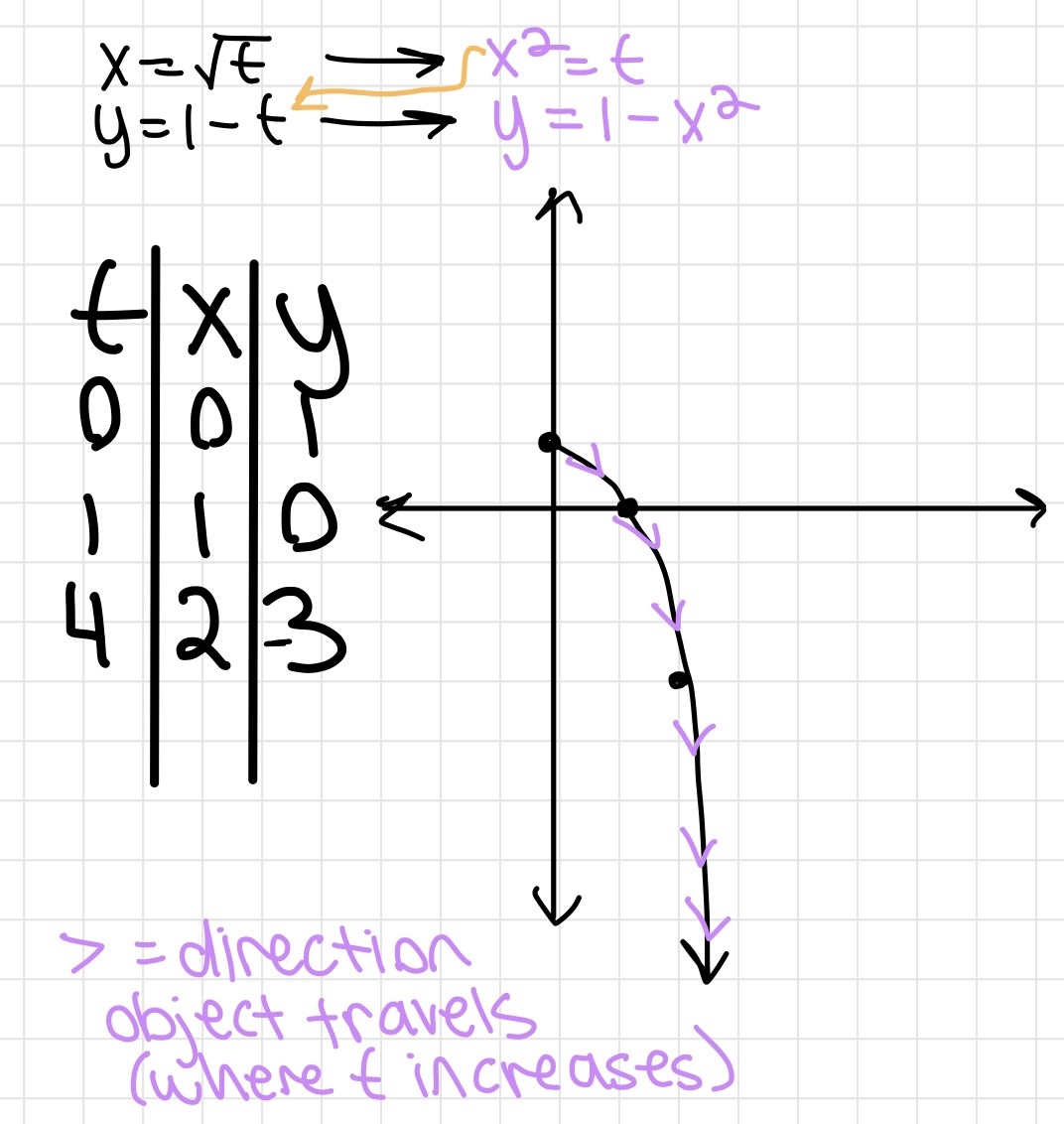
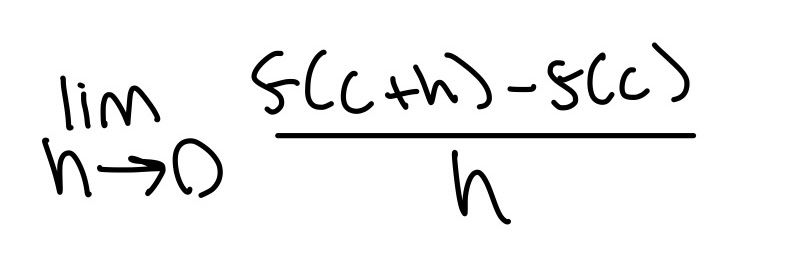Domains and Graphing Basics
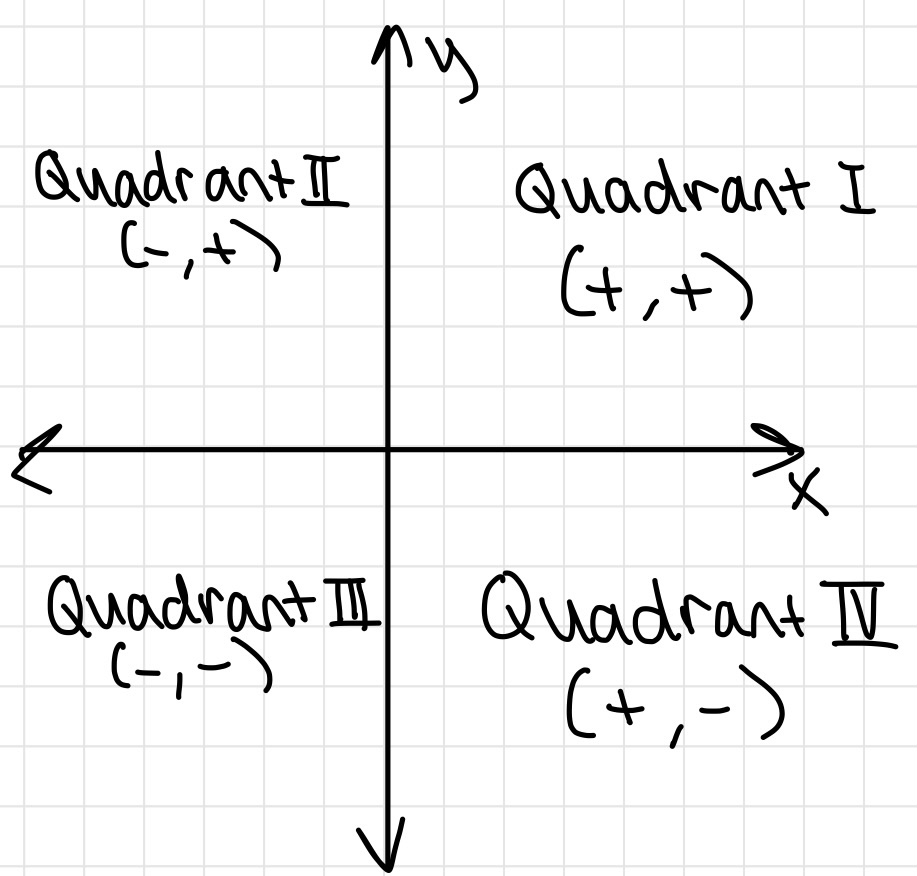
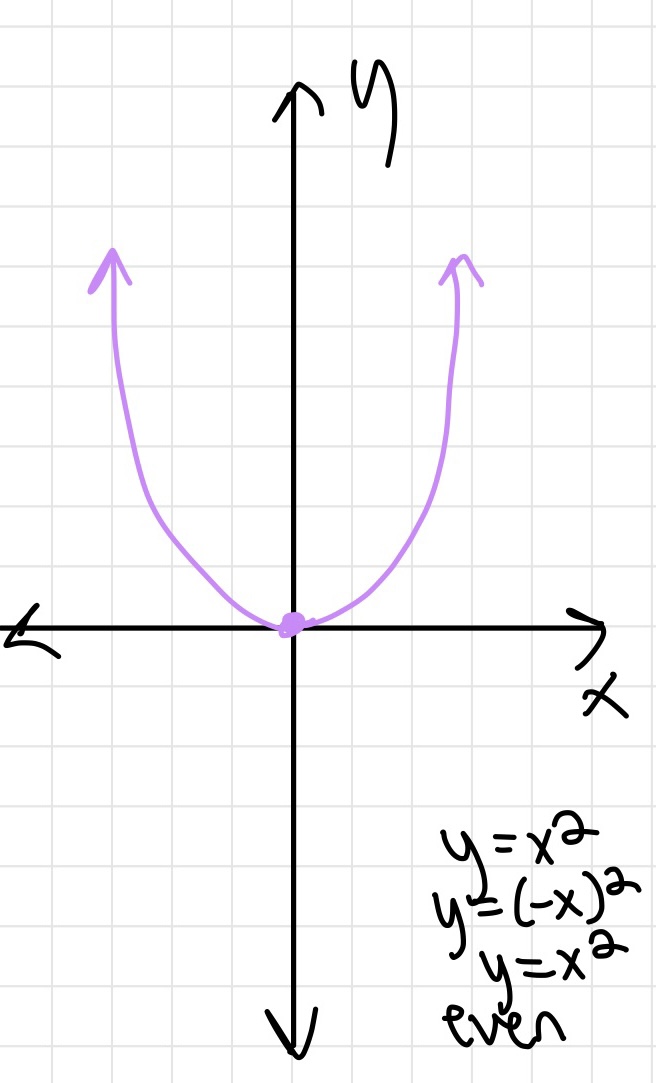
Coordinate Plane Quadrants
Precalculus is not as scary as it may seem. Do not worry. We got you :D
For the trigonometry formulas, please refer to the Trigonometry Page
Many concepts are repeated from Algebra 2, so they may seem familiar to you


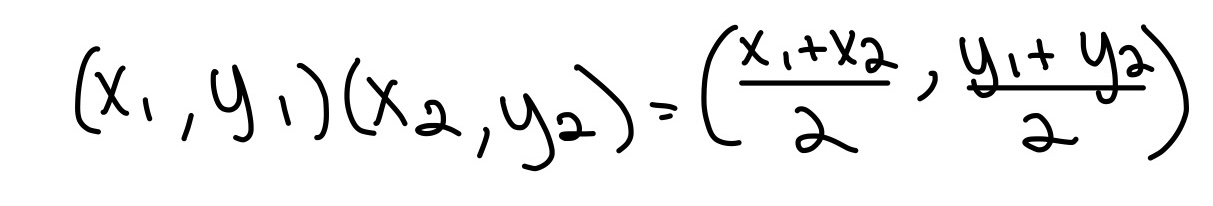


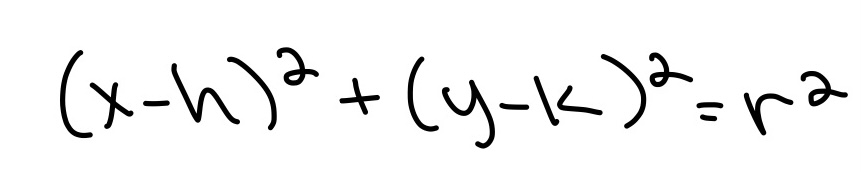

AKA average rate of change


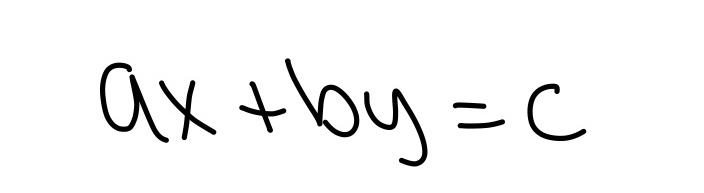



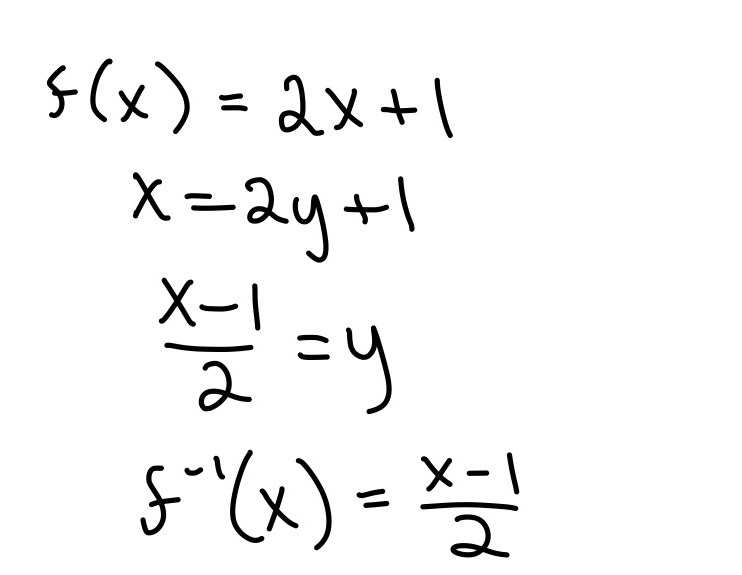
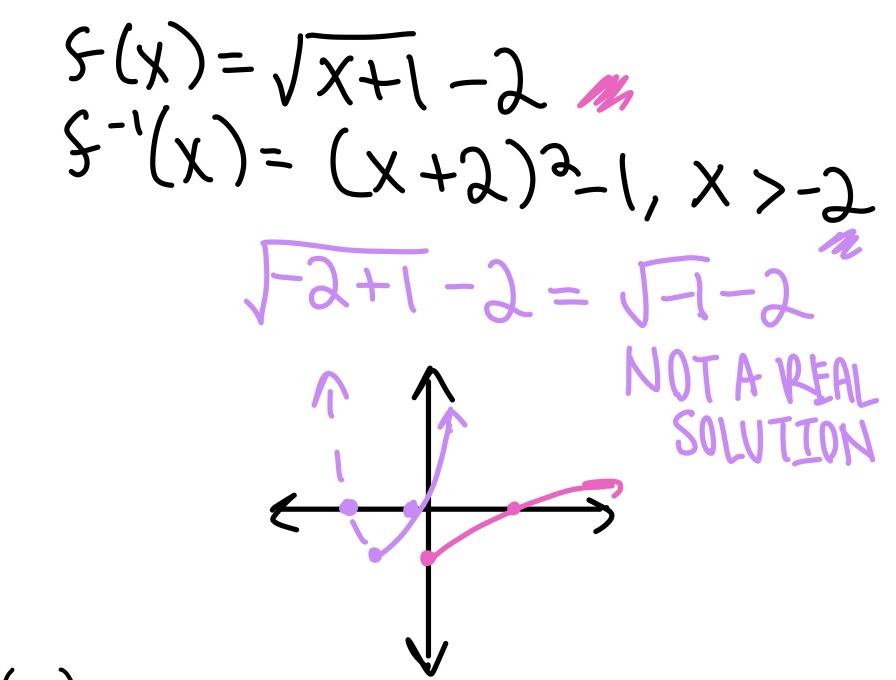
Ex. The inverse of f(x) = (√x + 1) - 2 is f(x) = (x+2) 2 - 1.
The restriction is x > -2. If x = -2, the original equation will be √-1 - 2 which is no solution
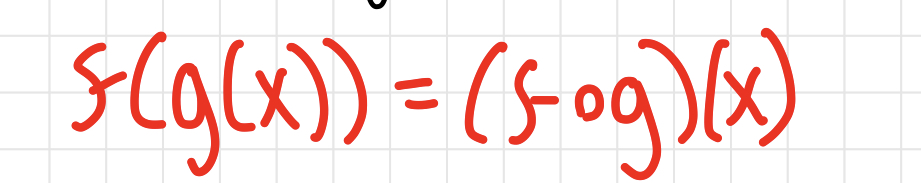


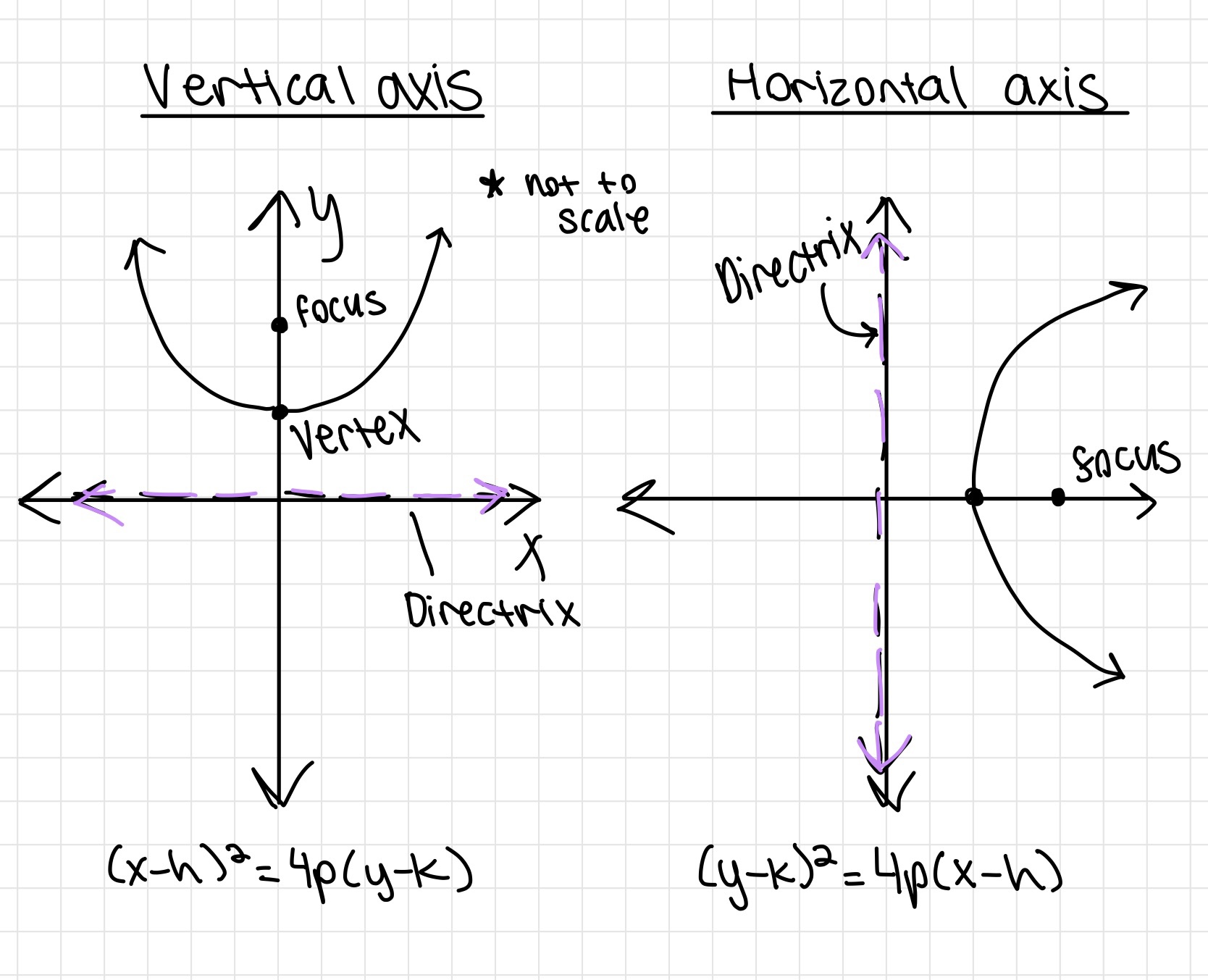
First equation is in quadratic form, second one is in vertex form



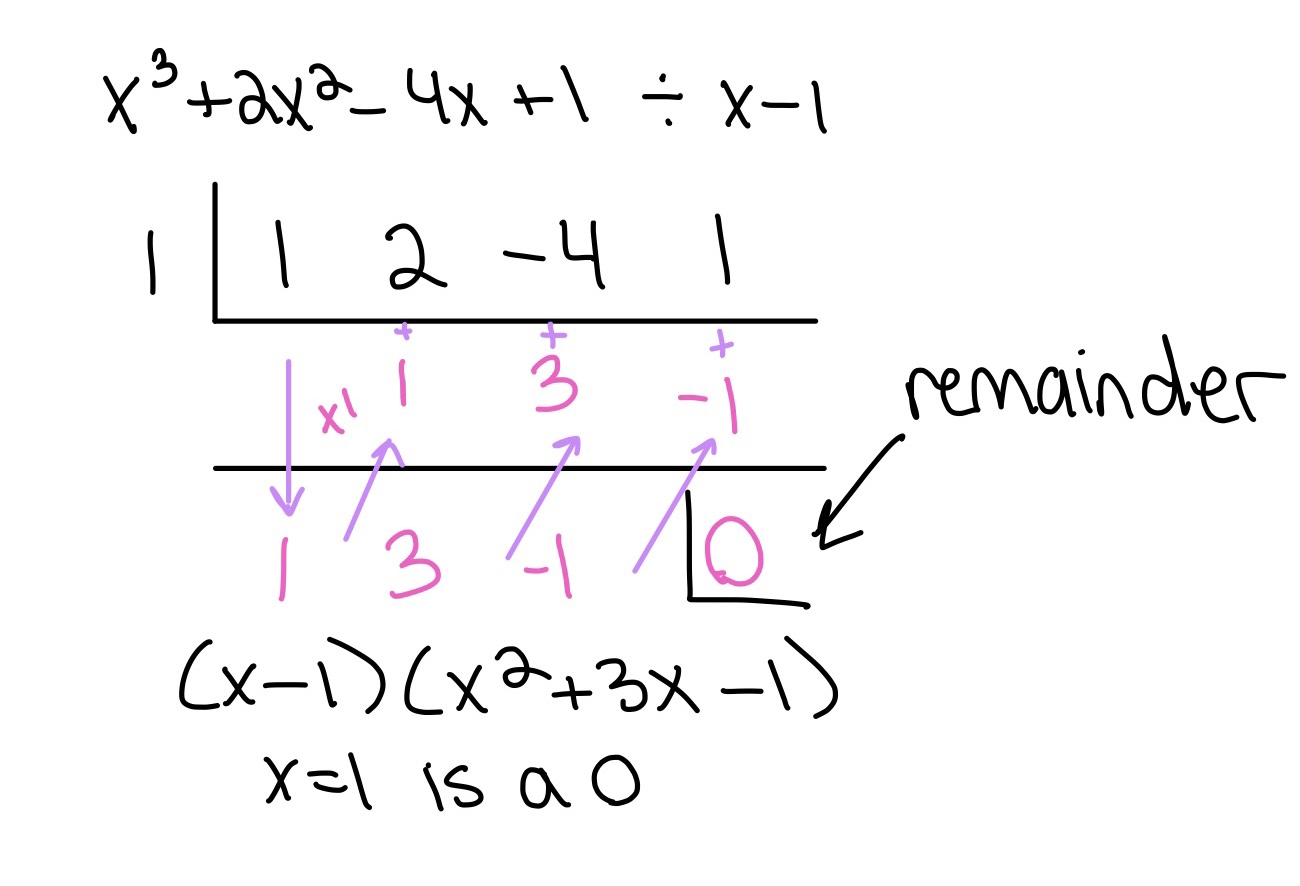

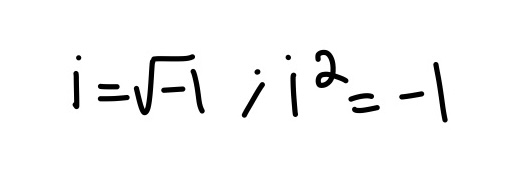


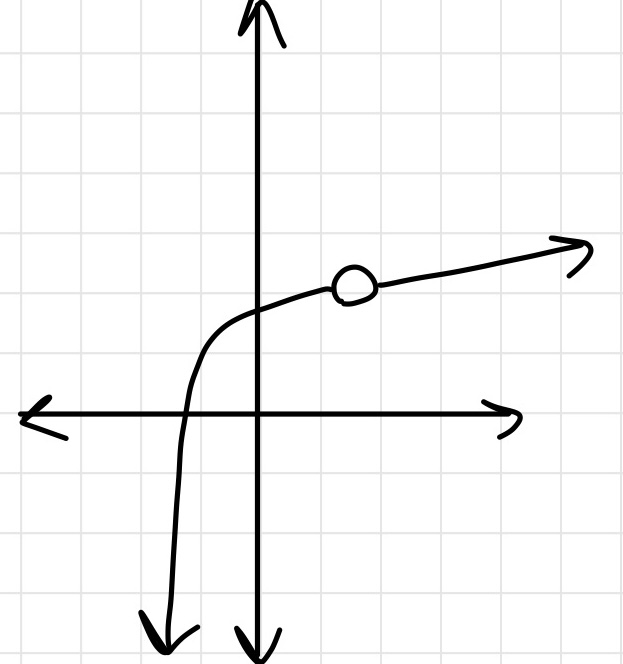


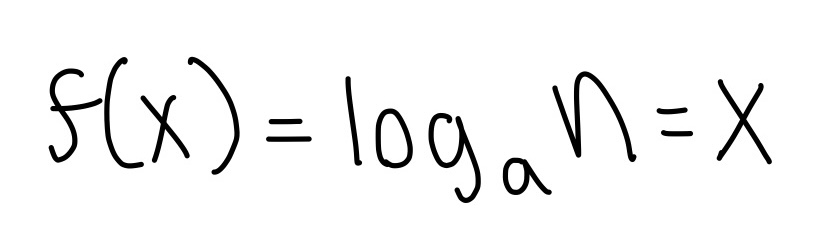
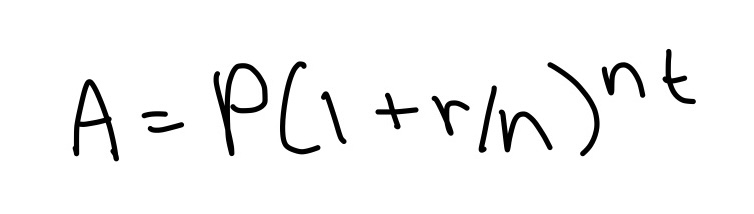

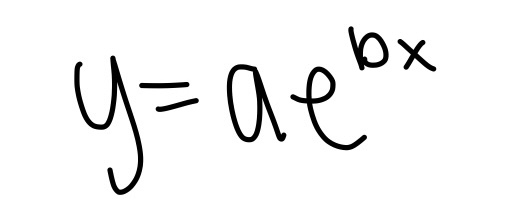
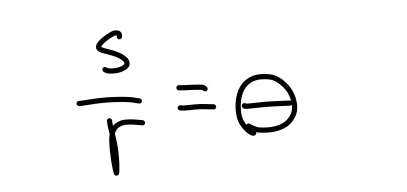
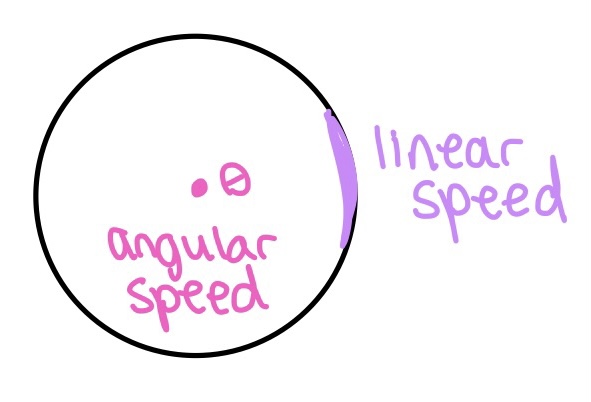


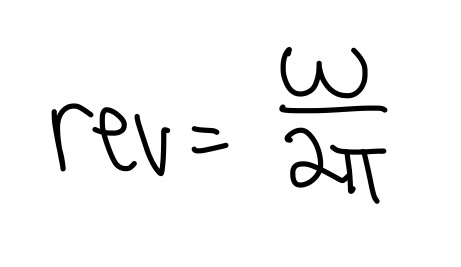
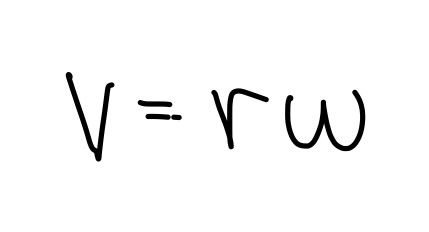

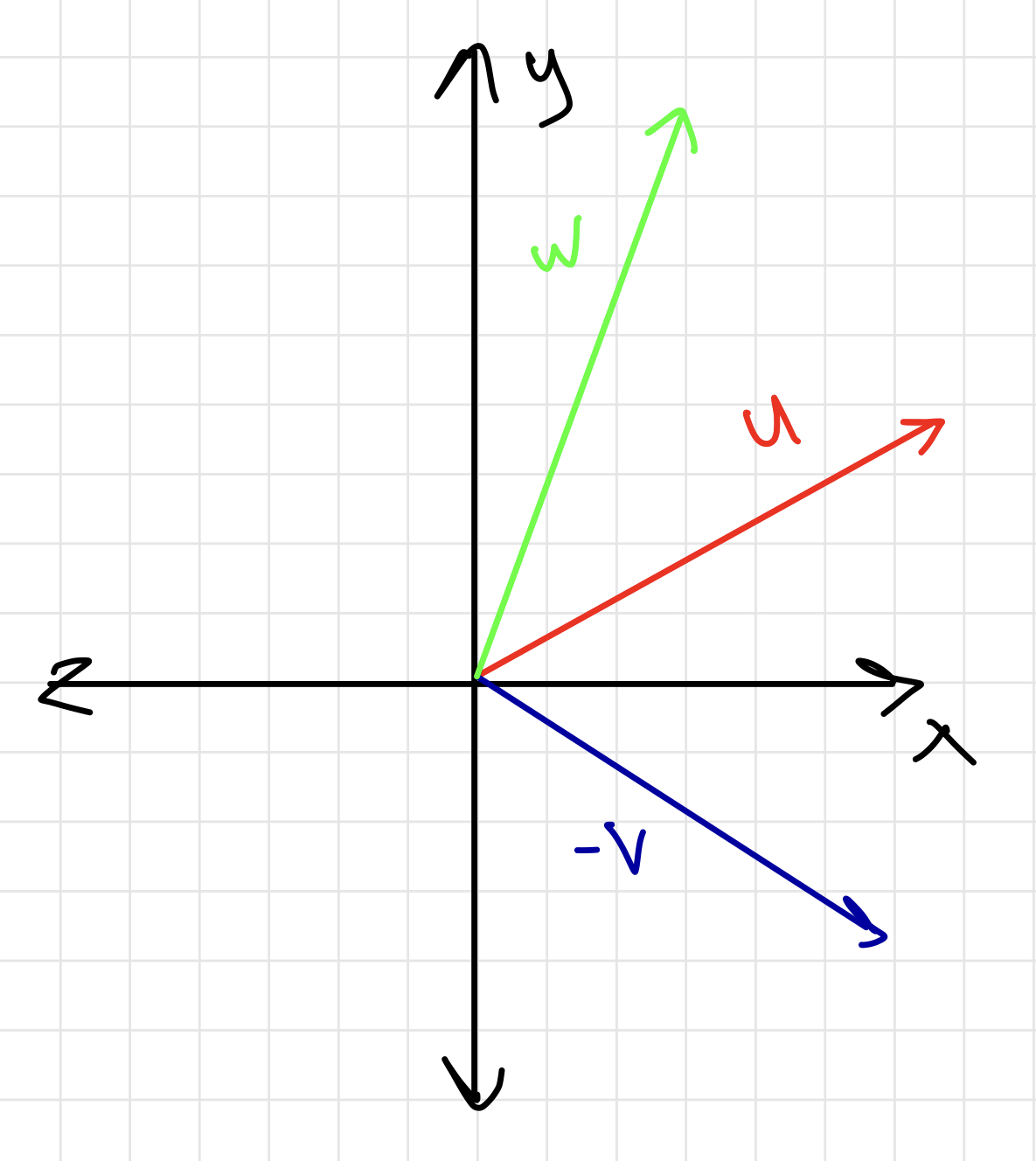
Click here for the distance formula


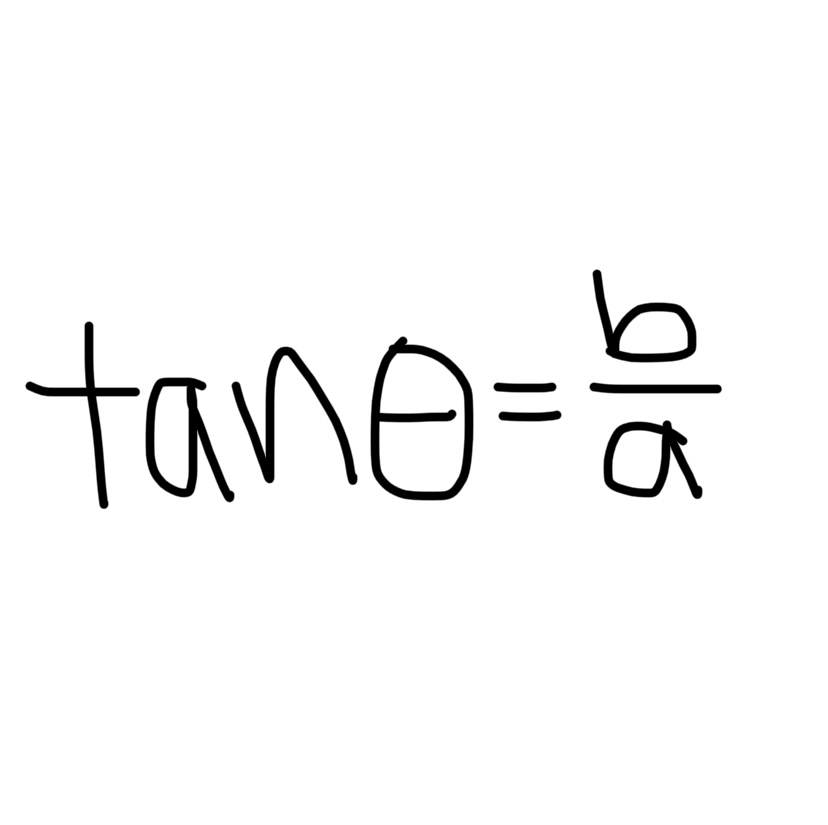
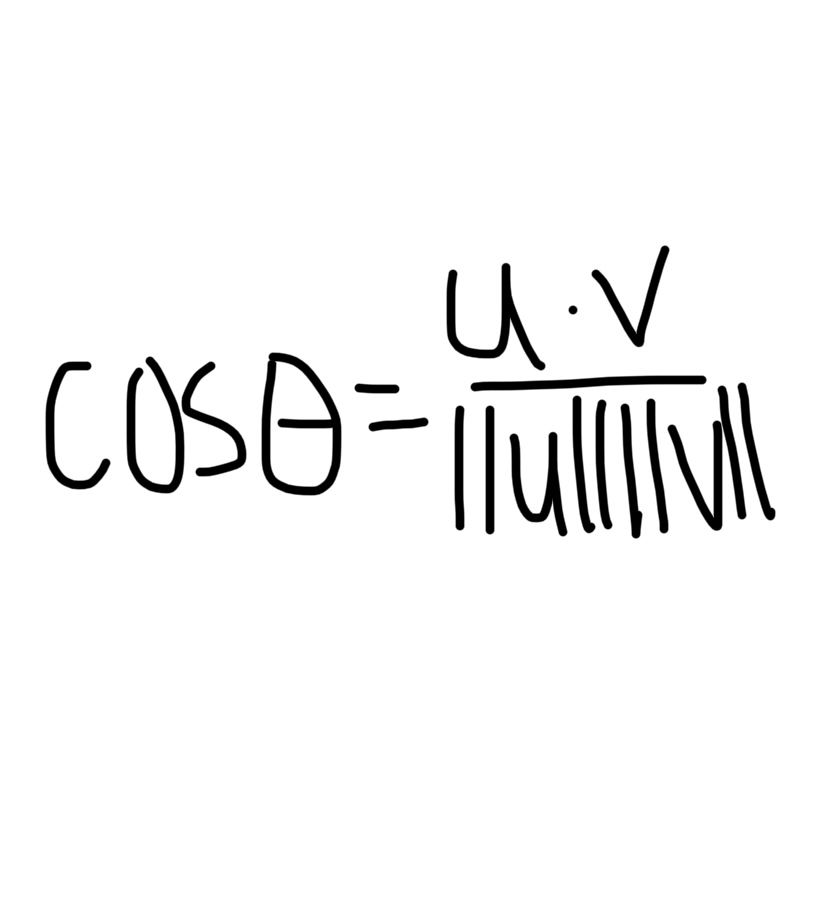
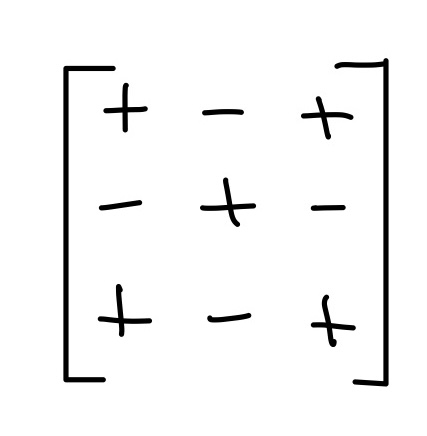
Matrix
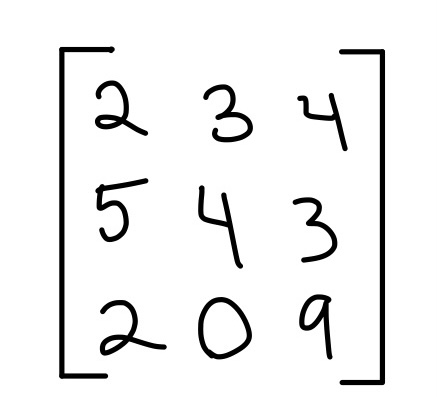
Augmented Matrix

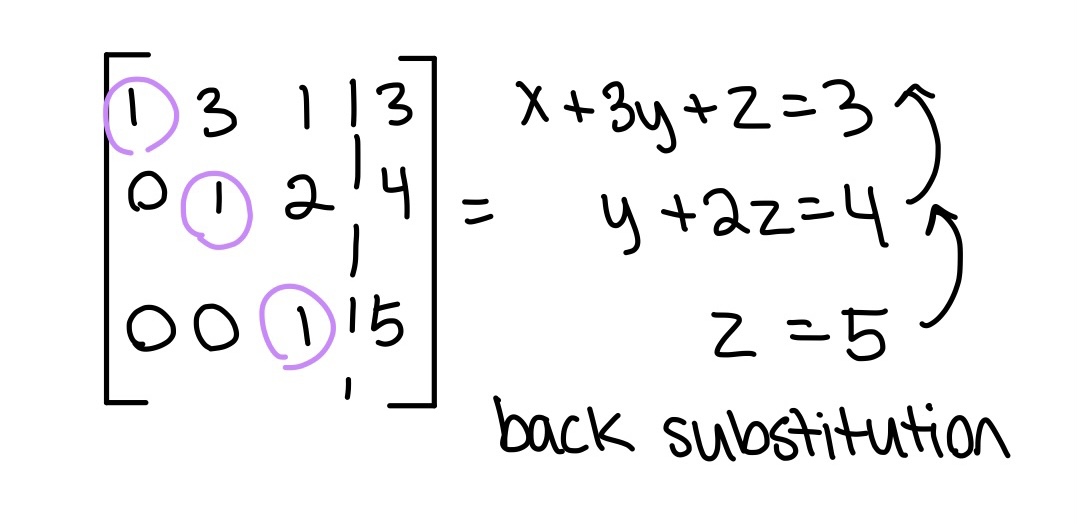
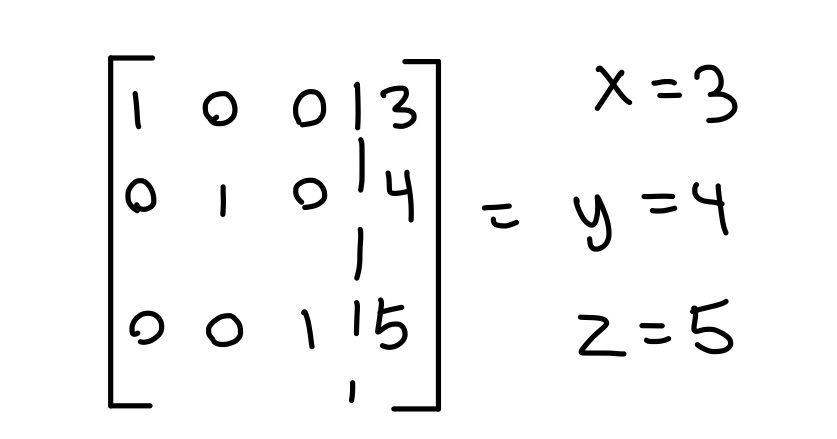

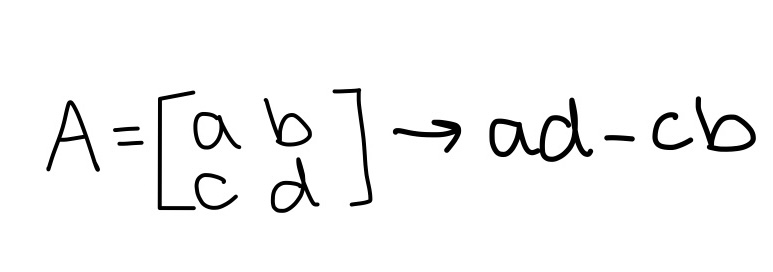

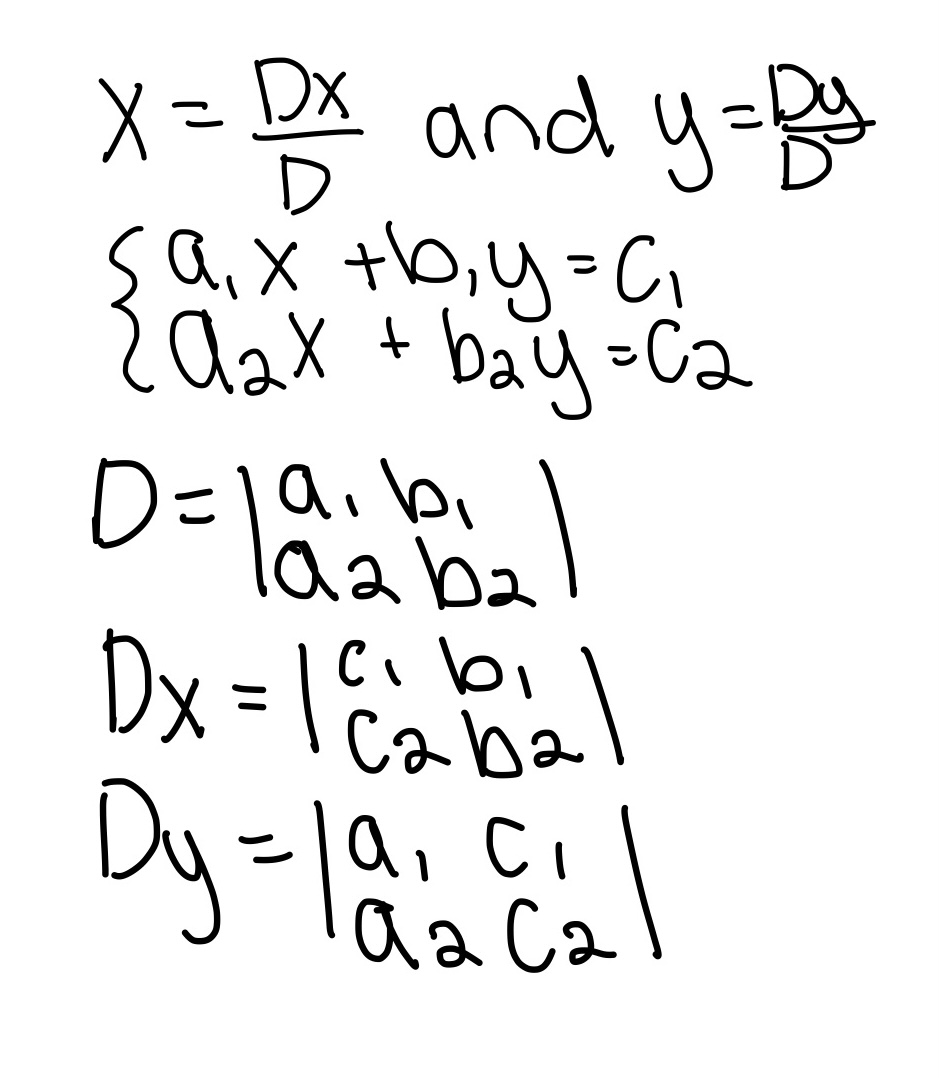
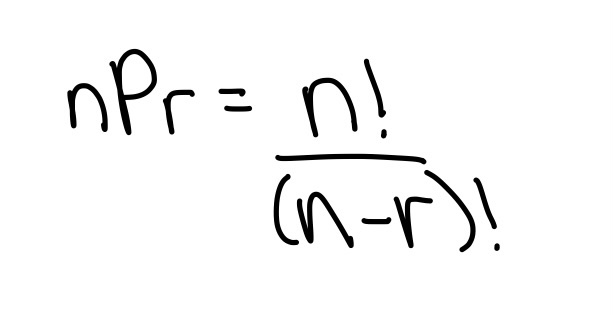
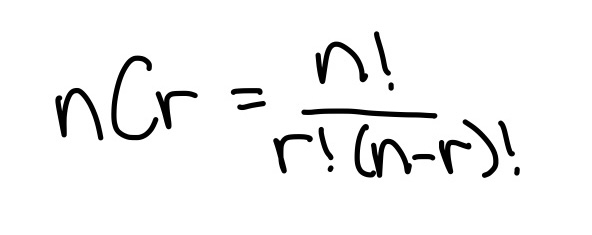

Use addition to solve the probability of the event.
Use multiplication to solve the probability of the event.
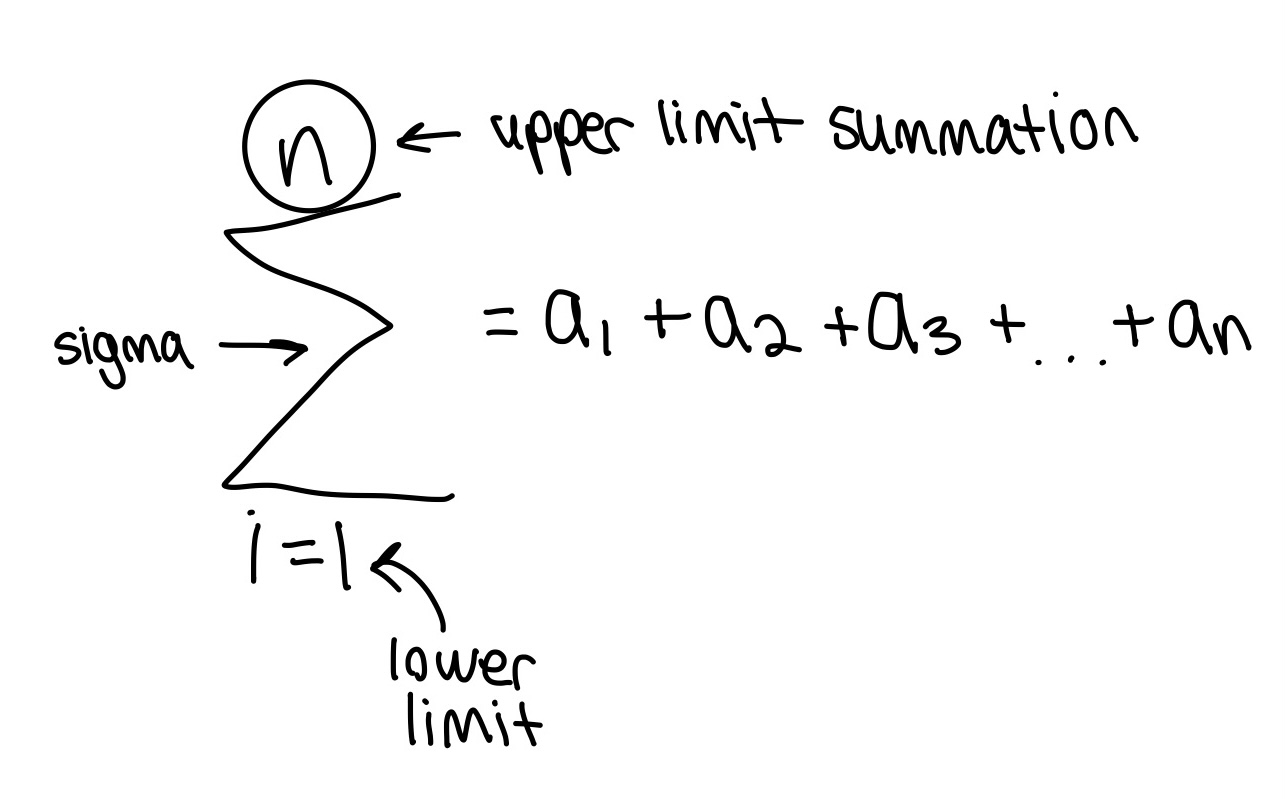
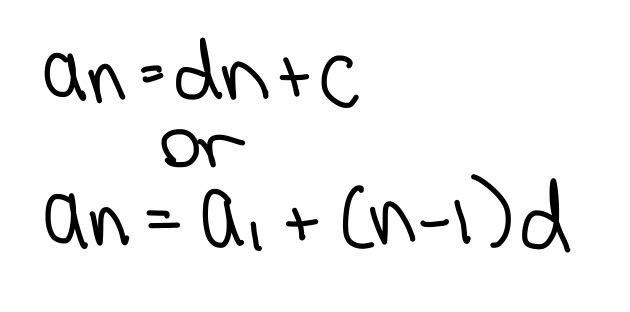

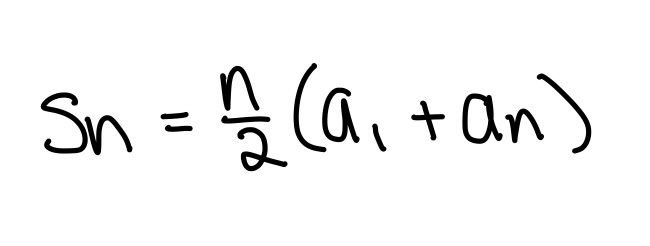
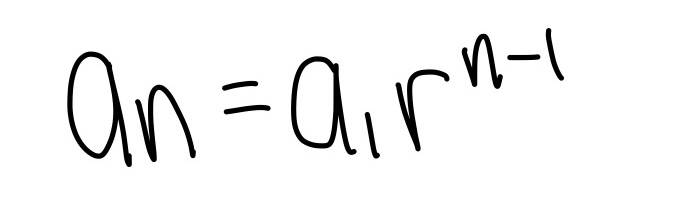

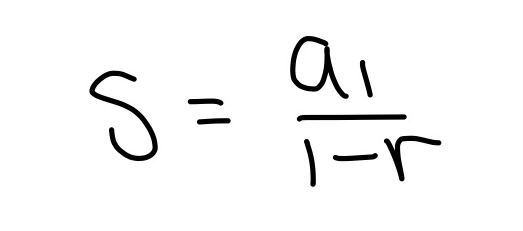

Used for calculus. Plug in the formulas below accordingly to solve the summation.

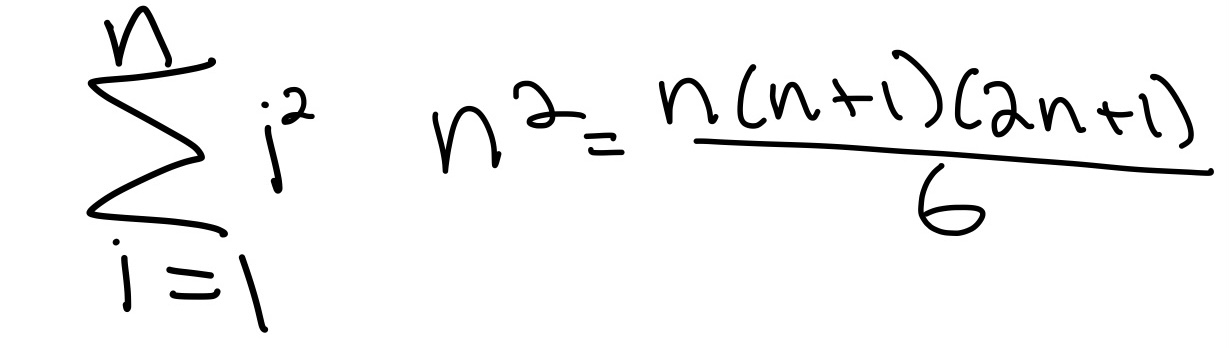



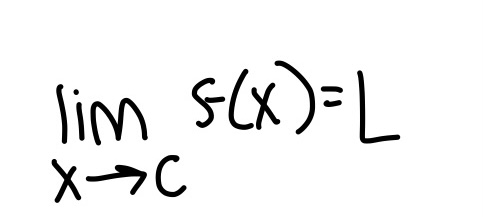

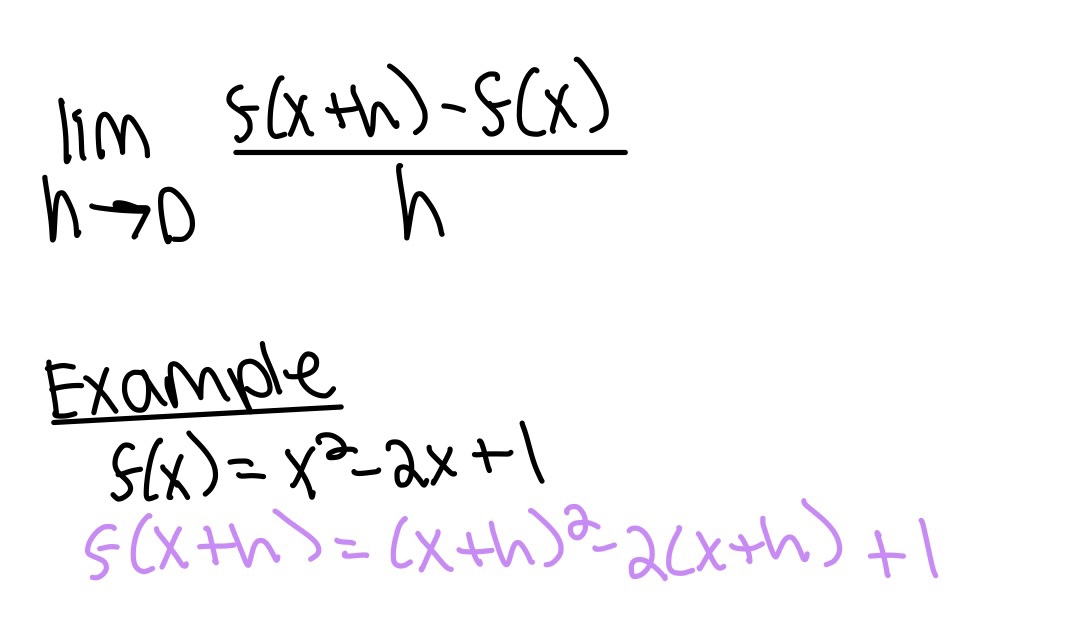
Definition of a derivative at a point x = c